





Abstract: To simulate quantitatively the microstructural evolution in the solidification process of multicomponent alloys, we extend the phasefield model for binary alloys to multicomponent alloys with consideration of the solute interactions between different species. These interactions have a great influence not only on the phase equilibria but also on the solute diffusion behaviors. In the model, the interface region is assumed to be a mixture of solid and liquid with the same chemical potential, but with different compositions. The simulation presented is coupled with thermodynamic and diffusion mobility databases, which can accurately predict the phase equilibria and the solute diffusion transportation in the whole system. The phase equilibria in the interface and other thermodynamic quantities are obtained using Thermo-Calc through the TQ interface. As an example, two-dimensional computations for the dendritic growth in Al-Cu-Mg ternary alloy are performed. The quantitative solute distributions and diffusion matrix are obtained in both solid and liquid phases.
Ⓒ2006 Acta Materialia Inc. Published by Elsevier Ltd. All rights reserved.
Key words : Phase-field models; Thermodynamics; Diffusion; Multicomponent solidification
The phase-field model is becoming a powerful tool which can describe complex interface pattern evolution. The phase-field models were originally developed for solidification of pure materials [1-3] and then extended to binary alloys [4-6] .However, many general industrial alloys, such as iron, steel and aluminum alloys, are all multicomponent alloys. It can be said that the application of the phase-field model for solidification to practical technology is closely linked to our ability to model microstructural development in multicomponent alloys.
Most of the models [7-10] describing the solidification of multicomponent alloys are based on the assumption that the solute diffusion behaviors of the components are independent of each other. The diffusion fields in multicomponent alloys are then given by the same mathematical functions as in binary systems. There are several models that can describe the solute interactions in multicomponent alloy solidification [11-13] ; however, they are limited to one-dimensional problems.
The aim of this work is to develop the phase-field model for dendritic solidification in multicomponent alloys with consideration of the solute interactions between different species. These interactions have a great influence not only on the phase equilibria but also on the solute diffusion behaviors. The simulation process presented is coupled with thermodynamic and diffusion mobility databases, which can accurately predict the phase equilibria and the solute diffusion transportation in the whole system. As an example, two-dimensional computations for the dendritic growth in Al-Cu-Mg ternary alloy are performed.
The total free energy of the system is defined as
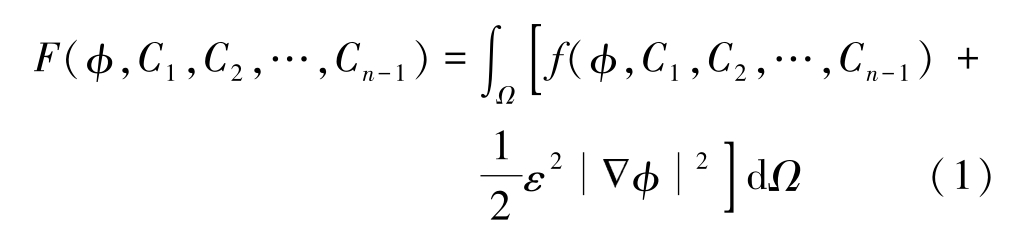
where F is the total free energy of the system, f is the free energy density, ε is the gradient energy coefficient and ϕ is the phase field ranging from zero in the liquid to one in the solid.
The governing equations for the phase field and the concentration field can then be expressed as [7]
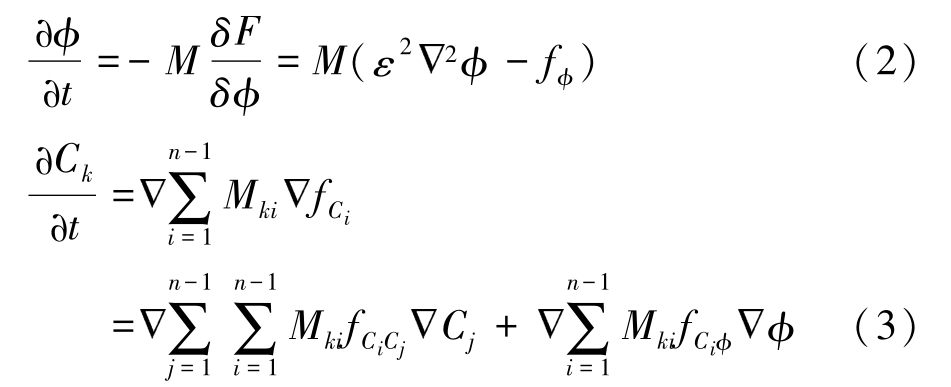
where f ϕ , f C , f Cϕ and f CC are ∂ f/ ∂ ϕ ,∂ f/ ∂ C i ,∂ 2 f/ ∂ C i ∂ ϕ and ∂ 2 f/ ∂ C i ∂ C j , respectively. M ki is determined from the diffusivity matrix:
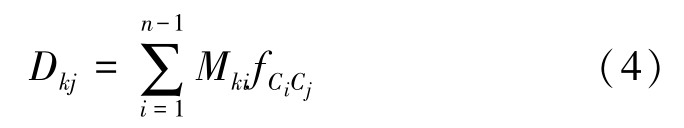
The diffusivity matrix in multicomponent alloys can be written as [14]
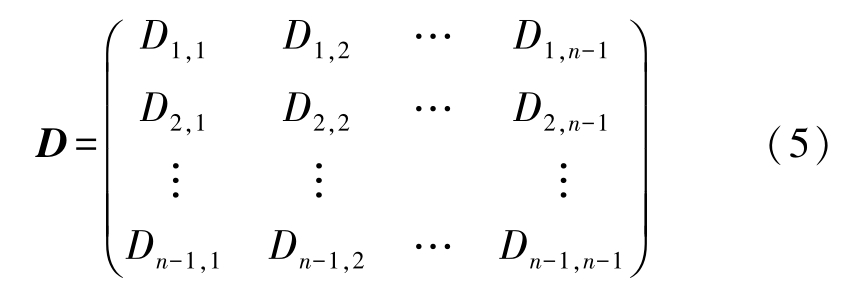
If the off-diagonal terms are set to zero, i. e.,

the solute diffusion transportation will be independent and can be determined by the same mathematical functions as in binary systems. In this paper, the diffusion matrix, both diagonal and off-diagonal terms, is accurately predicted by coupling with thermodynamic and diffusion mobility databases. This will be shown in Sec tion 3.2.
When the diffusivity matrix, D , is obtained, M ki can be determined by solving Eq. (4).
In this work, the free energy density is defined as [15]
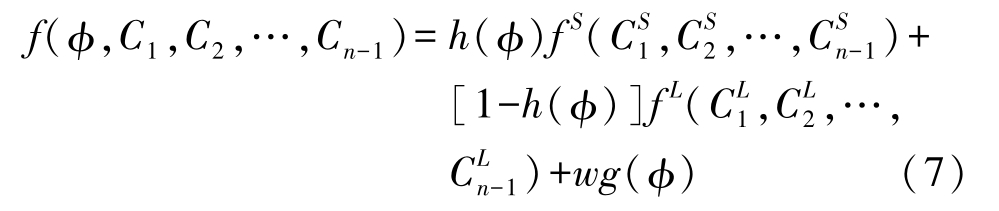
where
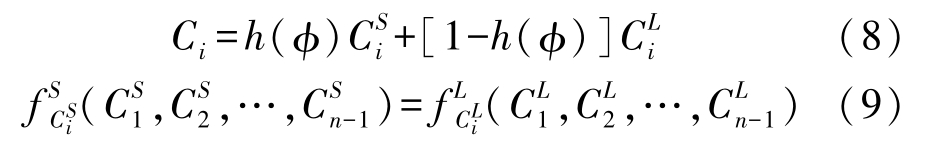
It is worth noting here that Eq. (9) is the equal chemical potential condition.
We choose:
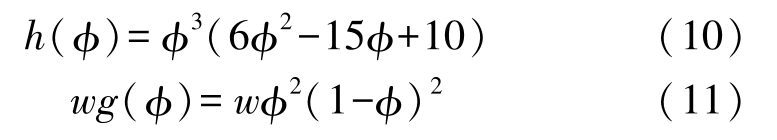
In the phase-field equation, there are three parameters:the mobility M , the gradient energy coefficient ε and the height of the parabolic potential w .the parameters w and ε can be obtained from the interface energy σ and the interface width 2 λ .Using the one-dimension equilibrium solution
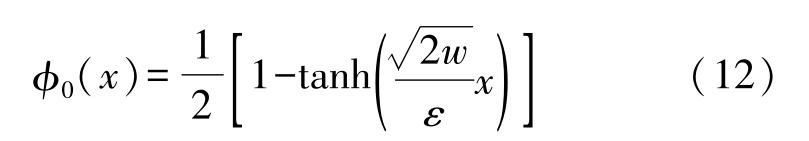
we can obtain the following relationships:
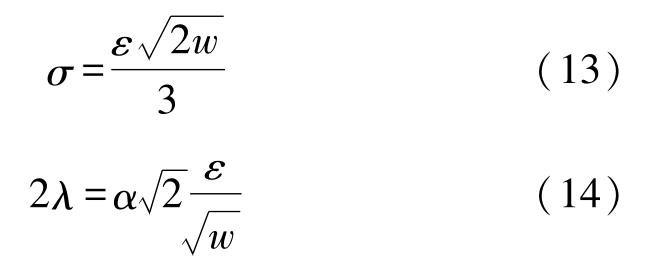
where α is a constant which is dependent on the definition of the interface thickness.
The phase-field mobility M is correlated with the interface kinetics and its equation is given as [6,7,15]
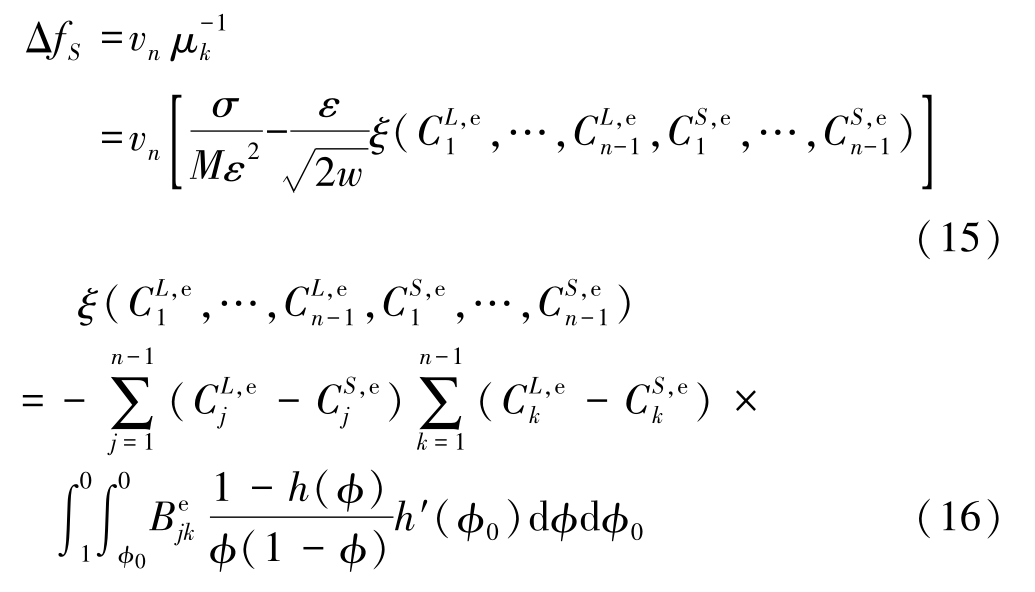
where
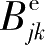 is a component of the inverse matrix of
is a component of the inverse matrix of
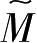 at the equilibrium state. If we apply a vanishing kinetic co efficient
[16]
, that is
at the equilibrium state. If we apply a vanishing kinetic co efficient
[16]
, that is
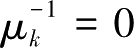 , the phase-field mobility can be expressed as
, the phase-field mobility can be expressed as
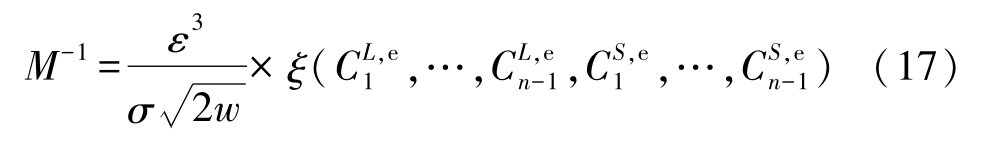
The present interface kinetics analysis differs from the analysis of Karma [17] , in which interface stretching and surface diffusion were considered.
In the case of multicomponent alloys, the integral Gibbs energy for each phase depends on the constitution, temperature and pressure, and this can be described by a thermodynamic model [18]
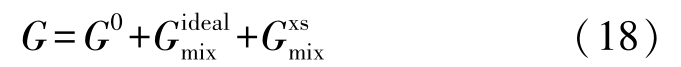
where
G
0
is the contribution of the pure components of the phase to the Gibbs energy,
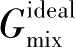 is the ideal mixing contribution and
is the ideal mixing contribution and
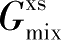 is the contribution due to non-ideal interaction between the components, also known as the Gibbs excess energy of mixing.
is the contribution due to non-ideal interaction between the components, also known as the Gibbs excess energy of mixing.
There are a large number of thermodynamic models for various substances in different states, such as the Redlich-Kister-Muggianu formalism [19] for face-centered cubic (fcc), body-centered cubic (bcc) and hexagonal closepacked (hcp) solid solution phases and the sublattice model [20] for the description of a phase with two or more sublattices. Such work has proved useful, since there are now several available software systems capable of estimating the phase equilibrium as a function of pressure, temperature and the composition of alloying elements, such as Thermo-Calc [21] , PANDAT [22] , MTDATA [23] , etc.
For the case that the elements are substitutional, the diffusion coefficient defined in the volume-fixed frame of reference,
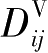 ,can be expressed as
[24]
,can be expressed as
[24]
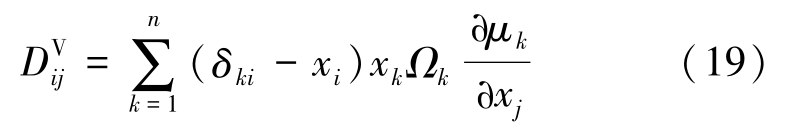
where x i is the mole fraction of component i , μ k is the chemical potential of component k and δ ki is the Kronecker delta, i. e., δ ki =1 when i = k and 0 otherwise. It can be noted that the quantity ∂ μ k / ∂ x j is a purely thermodynamic parameter and corresponds to the thermodynamic factor [25] .This quantity can thus be evaluated from the thermodynamic description of the system. The parameter Ω k is the mobility of species k in a given phase and will be discussed later.
By eliminating the concentration element n , the chemical diffusion coefficients can be deduced as

where
n
is the solvent and
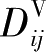 are given as in Eq. (19). The diffusivity
are given as in Eq. (19). The diffusivity
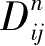 is the most convenient one for practi cal calculations. Determination the diffusion mobility parameter in Eq. (19) requires the use of experimental diffusion data. Tracer or self diffusivities,
is the most convenient one for practi cal calculations. Determination the diffusion mobility parameter in Eq. (19) requires the use of experimental diffusion data. Tracer or self diffusivities,
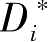 , are generally determined from diffusion studies using isotopes and directly related to the mobility
Ω
k
by means of the Einstein relation:
, are generally determined from diffusion studies using isotopes and directly related to the mobility
Ω
k
by means of the Einstein relation:

For the case that the elements are interstitial, the diffusion coefficient can be expressed as [24]

Using the diffusion coefficient expression in Eq. (20), the concentration of element n is eliminated and an ( n -1)×( n -1) chemical diffusion matrix is obtained.
For fcc, bcc and hcp solid solution phases and liquid phase, the Gibbs energy G for ternary alloys can be written as [18]
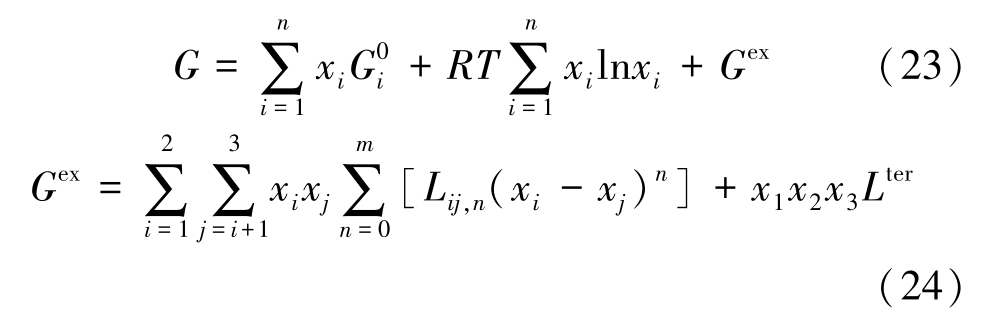
The thermodynamic description of the Al-Cu-Mg system from Buhler et al. [26] can now be used. Using the parameters for the thermodynamic description of Al-rich ternary Al-Cu-Mg alloys, G ex for liquid phase can be evaluated as
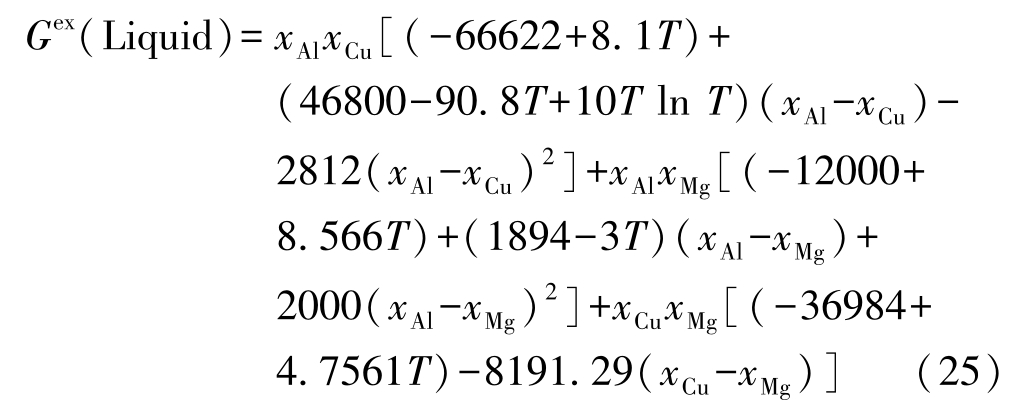
For fcc Al primary solution, G ex is given by
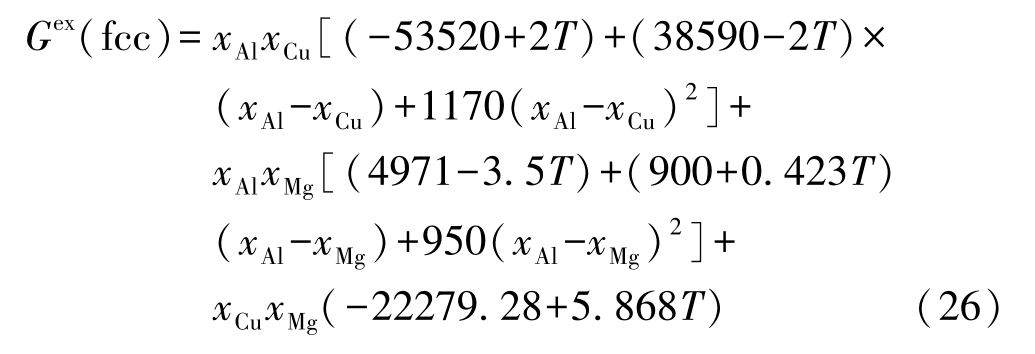
Tracer diffusion is the diffusion of a tracer in the matrix of an alloy. Tracer diffusivities are generally functions of composition. Here, we assume that the tracer diffusivities have no composition dependence. Assuming
x
is the concentration of tracer A, the tracer diffusivity
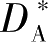 is equivalent to self-diffusion for
x
=1 and impurity diffusion for
x
→0. The impurity or self-diffu sivities,
is equivalent to self-diffusion for
x
=1 and impurity diffusion for
x
→0. The impurity or self-diffu sivities,
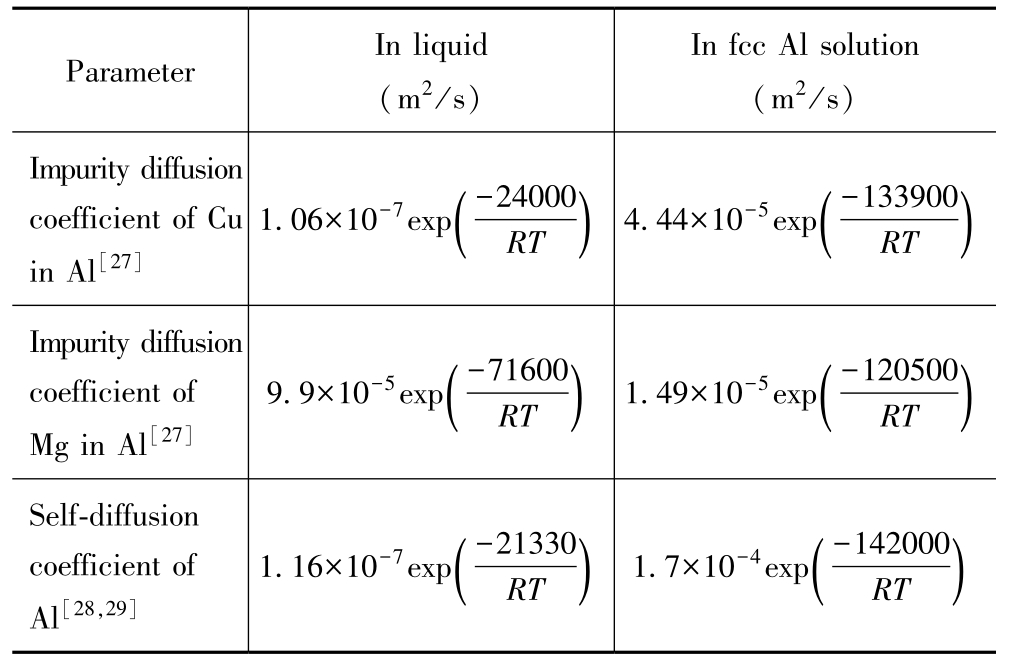
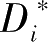 , in the ternary Al-Cu-Mg are given in Table 1.
, in the ternary Al-Cu-Mg are given in Table 1.
Table 1 Diffusion mobility data for Al-Cu-Mg alloy
Thus the phase equilibria in the solid-liquid interface can be obtained by the equal chemical potential criterion, i. e., Eq. (9). And the diffusion matrix for Al-Cu-Mg multicomponent alloys can be determined from Eqs. (19)~(22). The other parameters for Al-Cu-Mg alloys used in the simulation process are given in Table 2.
Table 2 Parameters used in the simulation
It is worth noting here that the phase equilibria and the thermodynamic factor in Eq. (19), ∂ μ k / ∂ x j , are obtained using Thermo-Calc [21] through the TQ interface.
Taking advantage of the four-fold morphological symmetry, only a quarter of the entire dendrite is chosen as the computational cell, and a solid seed is placed at the bottom-left corner, that is, at the origin. Equiaxed dendrite shape and the solute concentration fields for Al-2 at.% Cu-3.5 at.% Mg alloy are shown in Fig. 1 at the evolution time of 6×10 -5 s. It can be seen that primary stalk has a low concentration, but the regions between the secondary arms have the highest concentration. These features are commonly observed in real dendrites.
The diffusion matrix, both diagonal and off-diagonal items, is shown in Fig. 2.We can see that the diffusion coefficients are greatly concentration dependent. In the liquid phase, the absolute values of both diagonal and off-diagonal terms are larger at the interface than far from the interface, which correspond to the thick and dilute region, respectively. Some approximate analysis of the diffusion coefficients is given in Table 3.We can see that the ratio of off-diagonal to diagonal terms is approximately 10% for Cu and 15% for Mg.
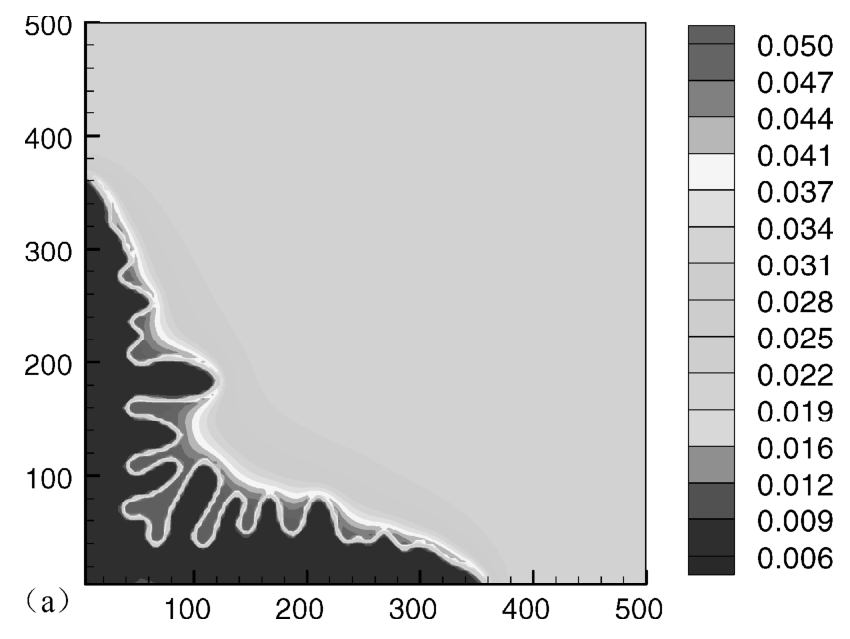
Fig. 1 Solute concentration profiles for the dendrite growth process of Al-2 at. % Cu-3.5 at. % Mg alloy at 900K( t =6×10 -5 s):(a) Cu, (b) Mg.
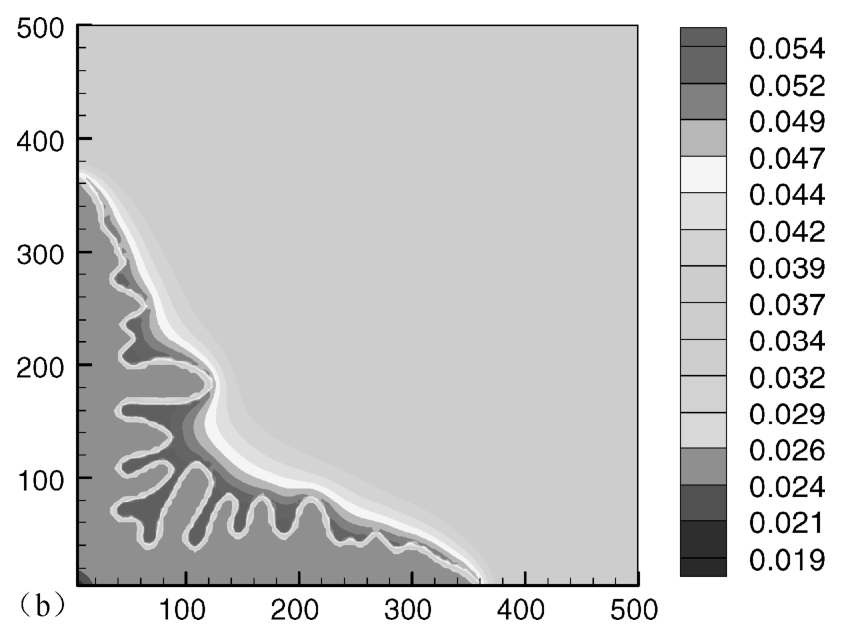
Fig. 1 Solute concentration profiles for the dendrite growth process of Al-2 at. % Cu-3.5 at. % Mg alloy at 900K( t =6×10 -5 s):(a) Cu, (b) Mg. (Continued)
In Fig. 2 we can also see that the off-diagonal diffusion coefficients are negative, which means that the solutes have attractive diffusional interaction [14] in Al-Cu-Mg alloy. If the off-diagonal diffusion coefficients are positive, there will be repulsive diffusional interactions between solutes in the system.
Table 3 Some approximate analysis ofthe diffusion coefficients
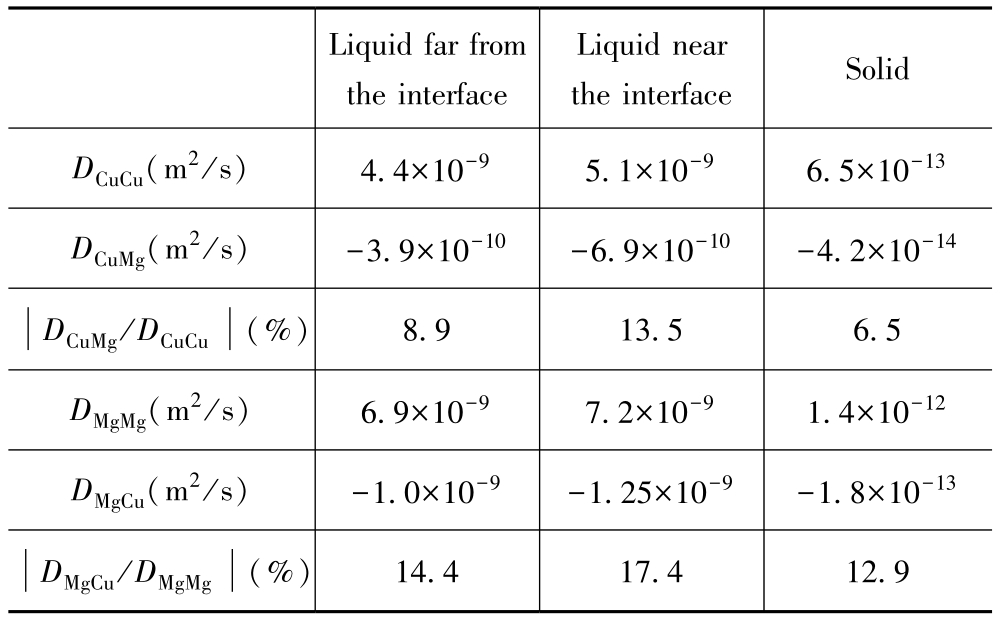
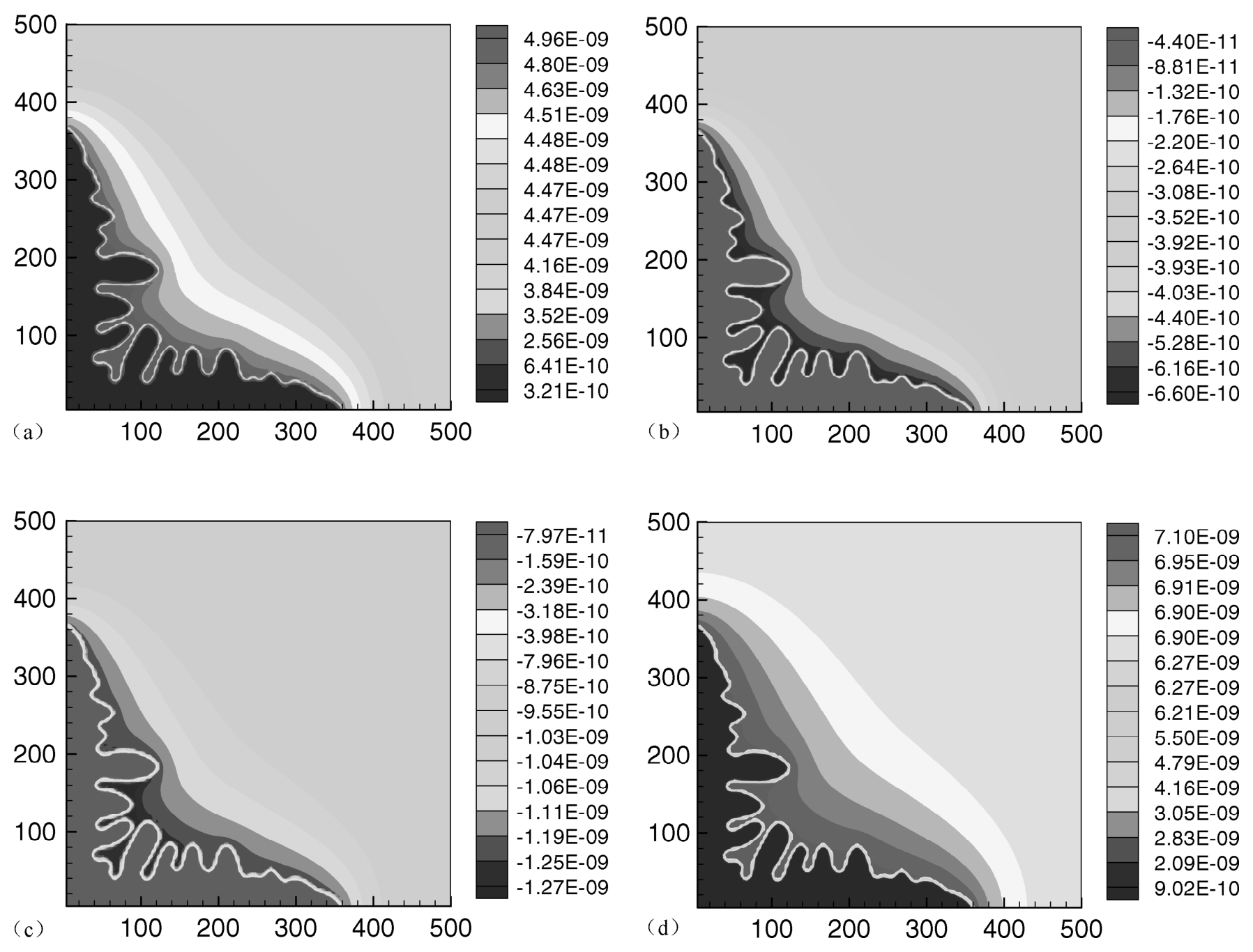
Fig. 2 Diffusion matrix profiles for the dendrite growth process of Al-2 at. % Cu-3.5 at. % Mg alloy at 900K( t =6×10 -5 s):(a) D CuCu , (b) D CuMg , (c) D MgCu , (d) D MgMg .
A phase-field model for solidification of multicomponent alloys is presented coupled with thermodynamic and diffusion mobility databases. By making direct use of the assessed thermodynamic and mobility databases, the phase equilibria and the solute diffusion transportation can be accurately predicted in the whole system. As an example, the two-dimensional computations for the dendritic growth in Al-Cu-Mg ternary alloy have been performed. The quantitative solute distributions and diffusion matrix are obtained in both solid and liquid phase. It is found that the diffusion coefficients are greatly concentration dependent. It is also demonstrated that this simulation can quantitatively model the isothermal dendrite growth in solidification of multicomponent alloys.
The study was financially supported by the National Significant Fundamental Research Project of MOST(2005CB724105) and the State Key Fundamental Research Project (G2000067202-1).
[1]Kobayashi R. Physica D 1993;63:3410.
[2]McFadden GB, Wheeler AA, Braun RJ, Coriell SR, Sekerka RF. Phys Rev E 1993;48:2016.
[3]Karma A, Rappel WJ. Phys Rev E 1999;60:3614.
[4]Wheeler AA, Boettinger WJ, McFadden GB. Phys Rev A 1992;45:7424.
[5]Karma A. Phys Rev E 1994;49:2245.
[6]Kim SG, Kim WT, Suzuki T. Phys Rev E 1999;60:7186.
[7]Cha PR, Yeon DH, Yoon JK. Acta Mater 2001;49:3295.
[8]Qin RS, Wallach ER. Acta Mater 2003;51:6199.
[9]Kobayashi H, Ode M, Kim SG, Kim WT, Suzuki T. Scripta Mater 2003;48:689.
[10]Sakai K. J Cryst Growth 2002;237-239:144.
[11]Chen Q, Ma N, Wu KS, Wang YZ. Scripta Mater 2004;50:471.
[12]Zhu JZ, Wang T, Zhou SH, Liu ZK, Chen LQ. Acta Mater 2004;52:833.
[13]Borgenstam A, Engström A, Höglund L,Ågren J. J Phase Equilib 2000;21:269.
[14]Hunziker O. Acta Mater 2001;49:4191.
[15]Cha PR, Yeon DH, Yoon JK. J Cryst Growth 2005;274:281.
[16]Ode M, Lee JS, Kim SG, Kim WT, Suzuki T. ISIJ Int 2000;40:870.
[17]Karma A. Phys Rev Lett 2001;87:115701.
[18]Saunders N, Miodownik AP. Calphad:a comprehensive guide. Pergamon: Oxford;1998.p.91.
[19]Peng M, Qiao Z, Mikula A. Calphad 1998;22:459.
[20]Hillert M. J Alloys Compd 2001;320:161.
[21]Andersson JO, Helander T, Höglund L, Pingfang Shi, Sundman B. Calphad 2002;26:273.
[22]Chen SL, Daniel S, Zhang F, Chang YA, Yan XY, Xie FY, et al. Calphad 2002;26:175.
[23]Davies RH, Dinsdale AT, Gisby JA, Robinson JAJ, Martin SM. Calphad 2002;26:229.
[24]Andersson JO, Agren J. J Appl Phys 1992;72:1350.
[25]Lupis CHP. Chemical thermodynamics of materials. Oxford: North-Holland.p.263.
[26]Buhler T, Fries SG, Spencer PJ, Lukas HL. J Phase Equilib 1998;19:317.
[27]Du Y, Chang YA, Huang BY, Gong WP, Jin ZP, Xu HH, et al. Mater Sci Eng A 2003;363:140.
[28]Cherne III FJ, Deymier PA. Scripta Mater 2001;45:985.
[29]Legzdina D, Parthasarathy TA. Metall Trans A 1987;18:1713.