





Single crystal superalloy turbine blades are currently widely used as key components in gas turbine engines. The single crystal turbine blade casting's properties are quite sensitive to the grain orientation determined directly by the grain selector geometry of the casting. A mathematical model was proposed for the grain selection during directional solidification of turbine blade casting. Based on heat transfer modeling of the directional withdrawing process , the competitive grain growth within the starter block and the spiral of the grain selector were simulated by using the cellular automaton method ( CA ). Validation experiments were carried out , and the measured results were compared quantitatively with the predicted results. The model could be used to predict the grain morphology and the competitive grain evolution during solidification , together with the distribution of grain orientation of primary < 001 > dendrite growth direction , with respect to the longitudinal axis of the turbine blade casting.
Currently, single crystal Ni-based superalloy turbine blades produced by Bridgman directional solidification technology are widely used in both aeronautic and energy industries as key components of the gas turbine engines. [1,2] The single crystal turbine blade casting's properties are quite sensitive to the grain orientation determined directly by the grain selector geometry of the casting. [3,4] Numerical simulation technology is used to study the directional solidification process and predict the final microstructure. [5,6]
In this paper, a mathematical model is proposed for the three-dimensional simulation of the directional solidification process of single crystal castings. Based on heattransfer modeling of the directional withdrawing process, the competitive grain growth within the starter block and the spiral of the grain selector (as shown in Figure 1) were simulated by using the cellular automaton method (CA). Validation experiments were carried out, and particular attention was paid to the grain morphology and grain orientation of primary <001> dendrite growth direction, with respect to the longitudinal axis of the turbine blade casting. The measured results were compared quantitatively with the predicted values.

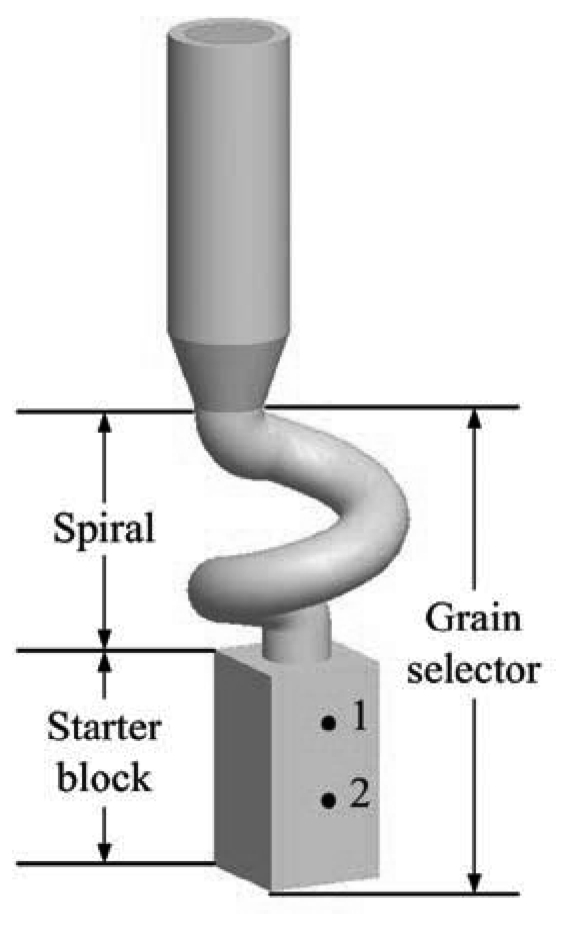
Fig. 1 Schematic of casting shape and thermocouple locations
As the foundation for the microstructure evolution simulation during directional solidification, the macro temperature distribution was calculated by the transient non-linear heat conduction equation as Equation (1), where T represents the temperature, t is the time, ρ is the density, c is the specific heat, L is the latent heat, λ is the heat conductivity, x , y and z are the coordinates; f S is the mass fraction of solid phase, Q R is the heat exchange between the casting surface and the ambient environment by radiation (Note that all equations are given in the table.)
It is difficult to calculate the heat radiation in directional solidification because of the memory required to store the view factors for each surface cell against others. In this paper, a numerical approach derived from Monte Carlo methods was adopted to deal with the radiation in directional solidification, with less memory required and higher accuracy. The details can be found in Reference[6].
The microstructure simulation was based on the modified CA method. [7] A continuous nucleation model [8] was employed to calculate the nucleus number in the undercooled melts as given in Equations (2) and (3);where Δ T is the undercooling, n (Δ T ) is the nucleus density, N max is the maximum nucleus density,Δ T σ is the standard deviation of the distribution,Δ T N is the average nucleation undercooling, f s (Δ T ′) is the fraction of solid phase.
Equations
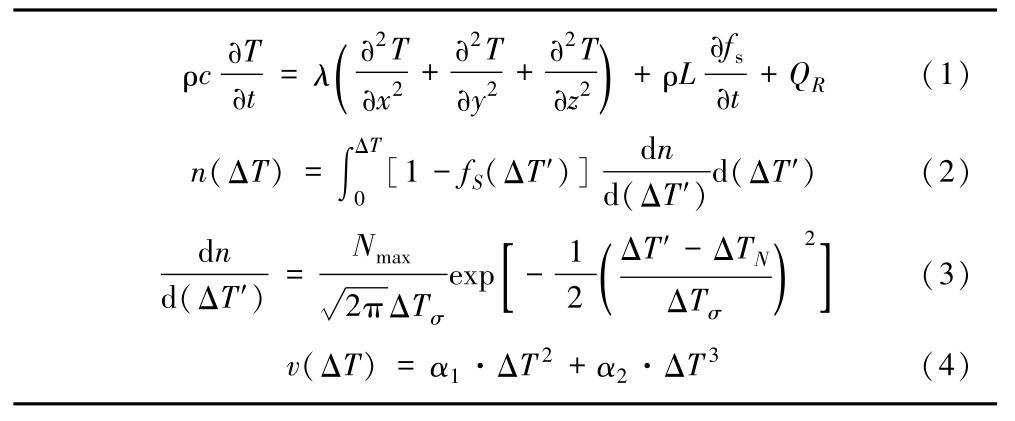
The grain growth was calculated based on the KGT model [9] with a simplification. [10] The growth speed of the dendrite tip was calculated as Equation 4; where α 1 and α 2 are the coefficients.
In this model, each grain's orientation was randomly determined. Nevertheless, the growth velocity of the grain tip was equally calculated by the temperature gradient in front of the dendrite tip. Therefore, the grains which were not well-aligned with respect to the maximum gradient of the temperature field would grow at a much slower speed than those which were best aligned, which made them grow behind the growth front. This made it possible for those well-aligned grains to have their secondary and tertiary side arms grow out and occupy the space just in front of the less well-aligned grains, as shown in Figure 2.This is known as the grain's competitive growth.
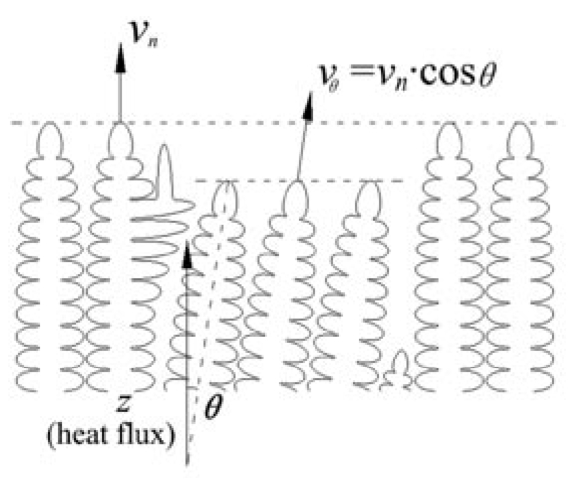
Fig. 2 Schematic of grain competitive growth.
Validation experiments of single crystal Ni-based superalloy casting were carried out. Cooling curves at different positions of the casting during directional solidification and the casting's final microstructures were measured and compared with the simulated results quantitatively. In the experiment, a group of three castings were produced together by the Bridgman directional solidification process, seen in Figure 3, for the schematic principle. The directional solidification furnace consists of a heating zone at the top, a cooling zone at the bottom, and a baffle in the middle to separate the heating and cooling zone, thus obtaining a steady high temperature gradient for the casting. At the beginning, the casting shell was placed on the top surface of the water-cooled chill plate, and heated by the heating zone for a certain time. After the melt metal was poured into theshell, the chill plate was withdrawn at a certain rate into the cooling zone. The withdrawal rate used in the experiment was 3.5mm/min. Thermocouples were placed on the surface of the casting for the temperature measurement. The casting shape and thermocouple locations are shown in Figure 1.The height of the casting is about 180mm.
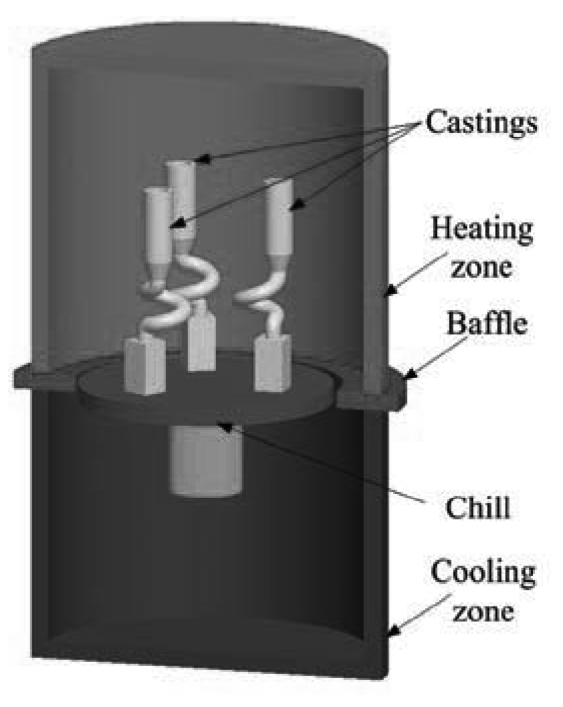
Fig. 3 Schematic of Bridgman directional solidification process.
The casting material used in the experiment was the DD6 Ni-based single crystal superalloy, nominal compositions [11] shown in Table 1.
Table 1 Nominal Compositions of DD6 Superalloy (wt.%)
Figure 4 shows the simulated and experimental cooling curves at point 1 and 2.The temperatures at both points dropped sharply after approximately 7 minutes from the beginning, and the simulated cooling curves at both points agreed with the experimental well.
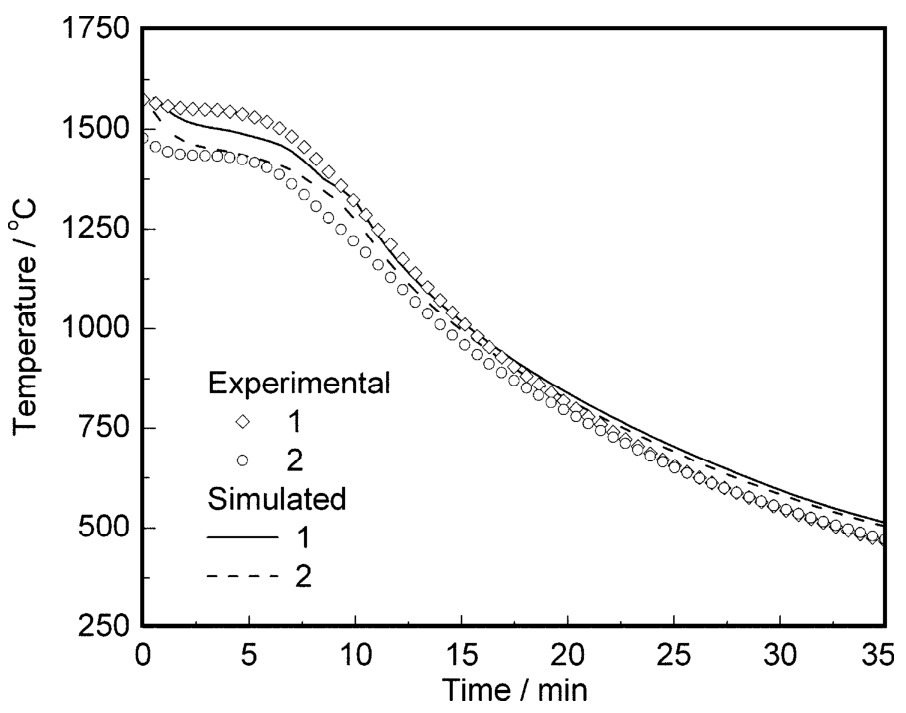
Fig. 4 simulated and experimental cooling curves.
Figure 5 shows the simulated and experimental microstructure of the starter block. The simulated microstructure had similar texture and grain sizes with the experimental microstructure.
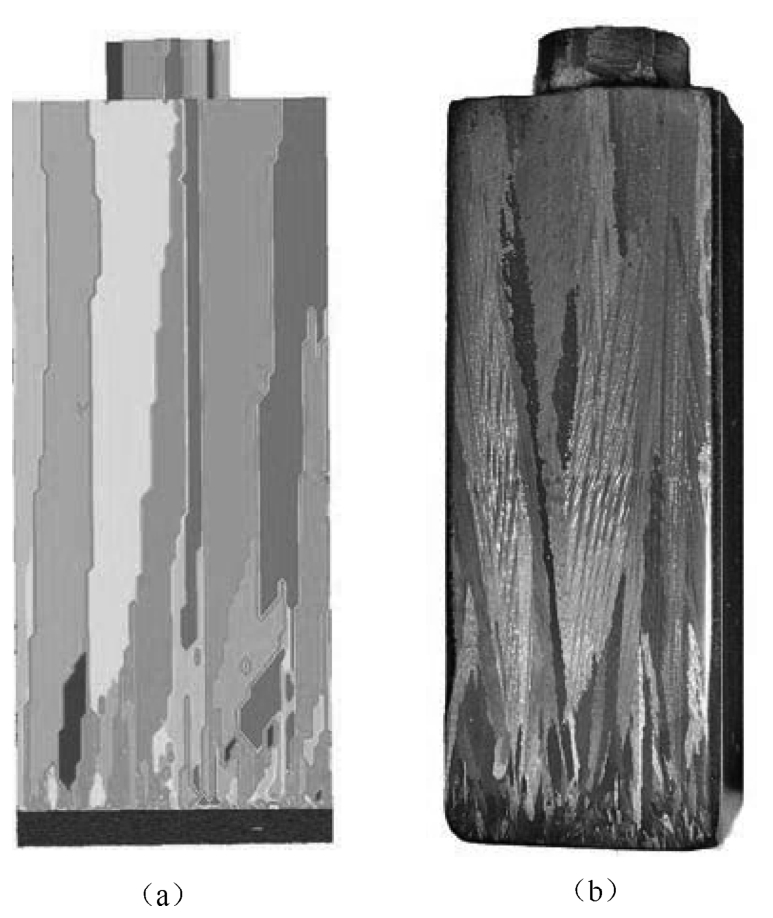
Fig. 5 (a) simulated and (b) experimental microstructure of the starter block.
Figure 6 shows the grain evolution process during solidification at different times. The liquid is not displayed so that the solid-liquid interface could be clearly shown. Figure 7 shows the simulated and measured grain number at different heights of the grain selector, in which the electron backscattered diffraction (EBSD) method was used for the experimental measurement of grain numbers at each transverse section. Good agreement was observed between the simulated and experimental results. It is indicated that a great number of tiny equiaxed grains emerged at the bottom surface of the starter block, and transferred into a much smaller number of columnar grains when growing upwards, which is well known as the grain competition process determined by the heat flux direction and the grain's fastest growth direction. The grain number decreased quickly in the starter block, leaving only less than 10 columnar grains growing into the spiral. The grains in the spiral continued to decrease when growing upwards, and only one of them survived from this grain selection, which finally grew into a whole single crystal casting.
Figure 8 shows the grain maps at different transverse sections of the grain selector, whose positions were previously indicated in Figure 6c. The results show that a great many small grains appeared at the bottom of the starter block and grew upwards. Only a few grains grew to the top of the starter block, which were allowed to grow into the spiral. In the spiral, the grains' growth was limited by the shape of the spiral, and only one of them survived in this selection. The simulated microstructure evolution results show that only one grain was left at the top of the casting, which initially nucleated at the bottom surface of the starter block and occupied the whole section in the middle circle of the spiral part.
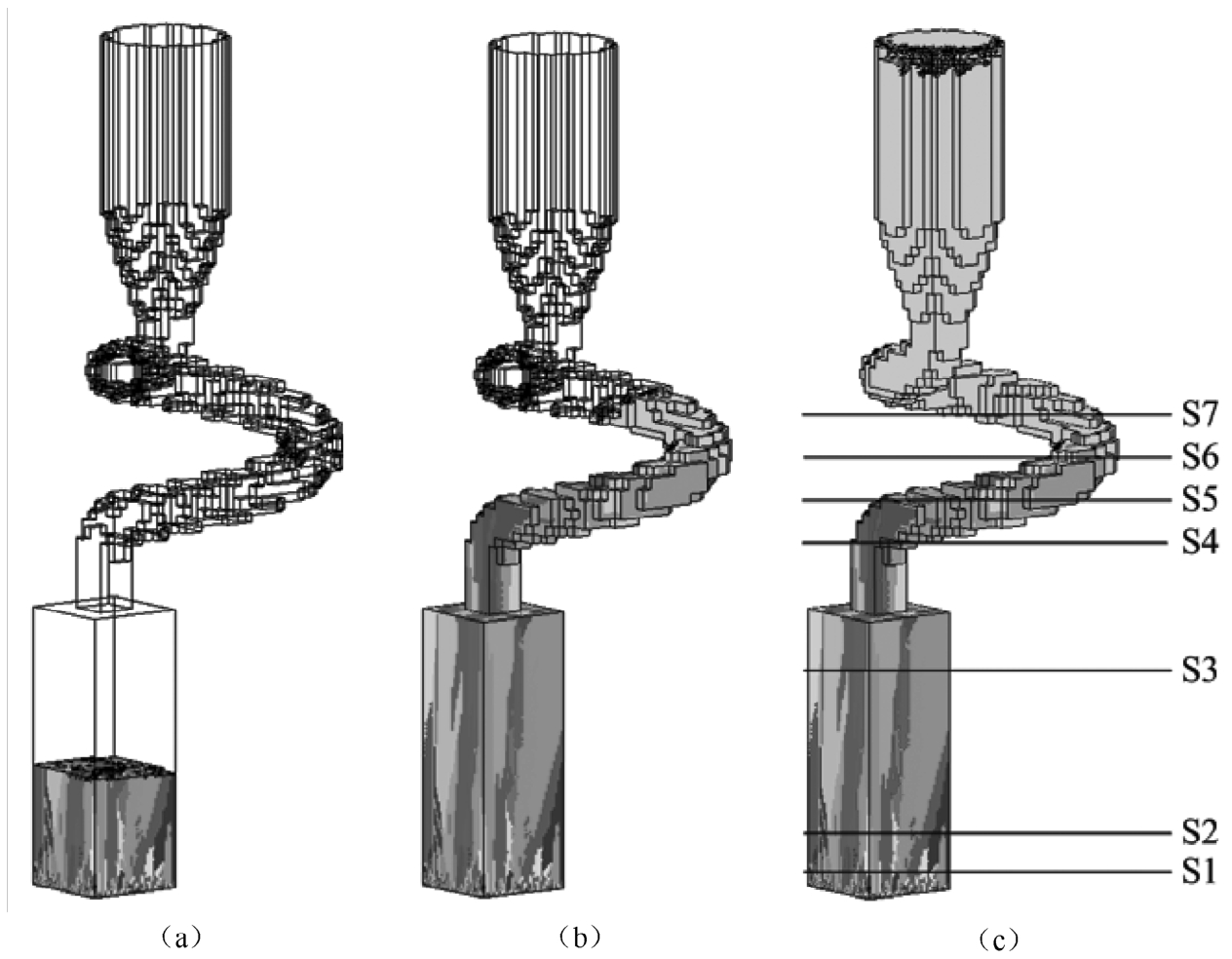
Fig. 6 Simulated grain evolution in the grain selector. (a) t =4.5min, (b) t =14.5min., (c) t =22.0min.
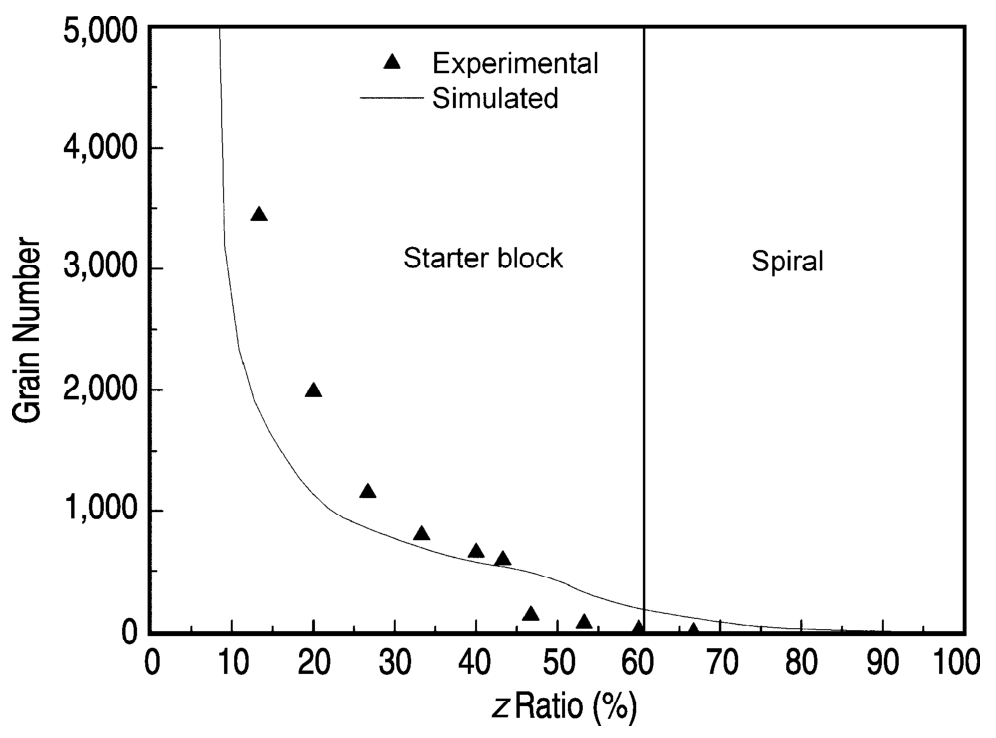
Fig. 7 Predicted and measured grain number at different positions of the grain selector.
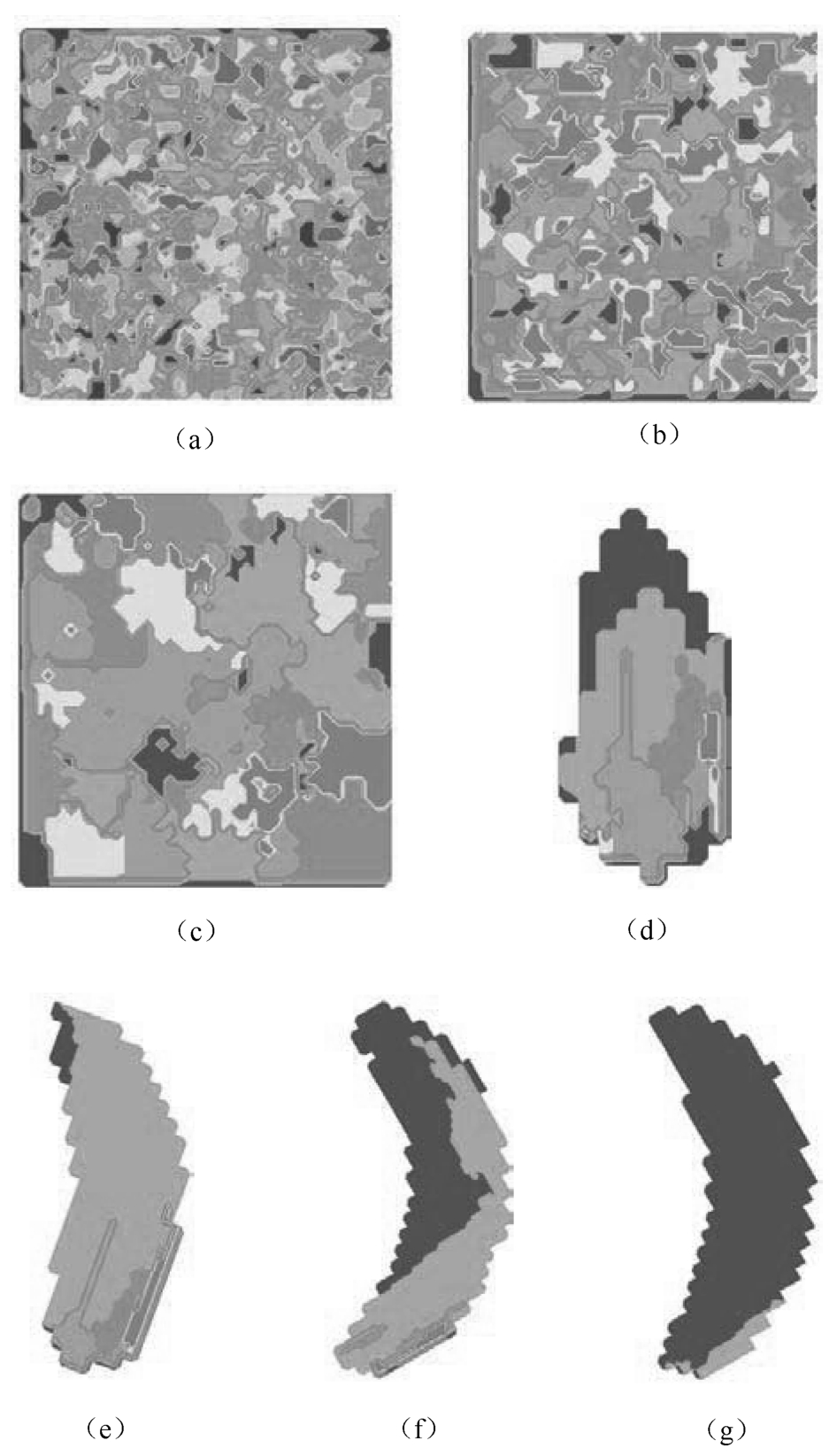
Fig. 8 Simulated grain maps of different transverse sections. (a) S1, (b) S2, (c) S3, (d) S4, (e) S5, (f) S6, (g) S7.
Figure 9 shows the comparison of predicted and measured variations of angle at several transverse sections in the starter block as indicated in Figure 6c, in which the angle is defined as the shortest angular rotation between the primary <001> direction of the grain and the normal of the chill surface. Both the simulated and experimental results show that the grain orientation near the bottom of the starter block was nearly random. Together with the decrease of the grain number causedby the competitive growth in the starter block, the grain orientation clustered into a smaller range of angle. At the end of the starter block, the grain orientation clustered into a narrow 5°~15° range, showing the grain selection results caused by the competitive grain growth in the starter block. The predicted results show a reasonable correspondence to the measured results.
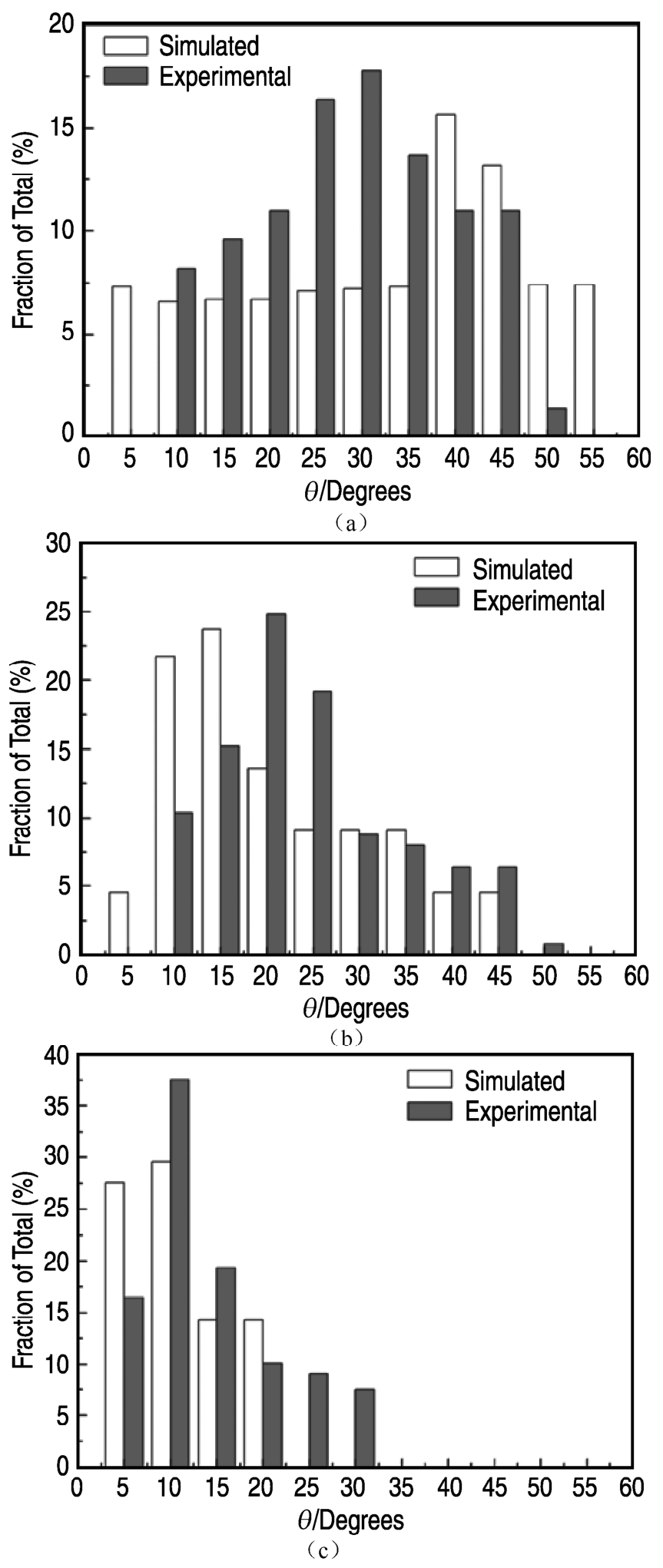
Fig. 9 Predicted and measured variations of grain orientation angle. (a) S1, (b) S2, (c) S3.
(1) A mathematical model was proposed for the three-dimensional simulation of grain selection during directional solidification of Ni-based single crystal superalloy turbine blade casting. Based on heat transfer modeling of the directional withdrawing process, the competitive grain growth within the starter block and the spiral were simulated by using cellular automaton method (CA).
(2) The simulated cooling curves and microstructures agree well with the experimental results. Only a few grains survived from the grain competition in the starter block and grew into the spiral, while just one of them was left at the middle height of the spiral and grew into a whole single crystal casting.
(3) The simulated grain orientation results of primary<001> dendrite with respect to the longitudinal axis of the turbine blade casting were compared quantitatively with the measured results, which shows a reasonable correspondence.
The work reported has been carried out under the financial support of National Basic Research Program of China ( No. 2005CB724105 ), National Natural Science Foundation of China ( No. 10477010 ), and National High Technology Research and Development Program of China ( No. 2007AA04Z141 ).
[1]R. C. Reed, The Superalloys Fundamentals and Applications (New York: Cambridge University Press,2006) pp.18-29.
[2]R. E. Schafrik and Scott Walston"Challenges for High Temperature Materials in the New Millennium, " Proceedings of the 11th International Symposium on Superalloys , ed. R. C. Reed et al. (Warrendale,PA: TMS,2008), pp.3-9.
[3]H. Esaka, K. Shinozuka, and M. Tamura, "Analysis of Single Crystal Casting Process Taking Into Account the Shape of Pigtail, " Materials Science and Engineering , A413-414(2005), pp.151-155.
[4]Z. G. Zhong, "Making Method of Controlled Single Crystal Seed (in Chinese), " Material Engineering , 9(1996), pp.39-41.
[5]P. Carter et al., "Process Modelling of Grain Selection during the Solidification of Single Crystal Superalloy Castings, " Materials Science and Engineering ,A280(2000), pp.233-246.
[6]J. Yu et al., "Numerical Simulation of Solidification Process on Single Crystal Ni-based Superalloy investment Castings, " Journal of Materials Science and Technology , (23)2007, pp.47-54.
[7]M. Rappaz and C. A. Gandin,"Probabilistic Modelling of Microstructure Formation in Solidification Processes, " Acta Metallurgica et Materialia , 41(1993), pp.345-360.
[8]P. Thevoz, J. L. Desbiolles, and M. Rappaz, "Modeling of Eguiaxed Microstructure Formation in Casting, " Metallurgical Transactions A , 20A(1989), pp.311-322.
[9]W. Kurz, B. Giovanola, and R. Trivedi. "Theory of Microstructure Development during Rapid Solidification, " Acta Metallurgica , 34(1986), pp.823-830.
[10]J. Lacaze, G. Lesoult."Effects of Solidification-conditions on Austenitic Stainless-steel Solidification-mode, " Journal of Crystal Growth ,89(1988),pp.531-544.
[11]J. R. Li et al., "Effects of Low Angle Boundaries on the Mechanical Properties of Single Crystal Superalloy DD6, " Proceedings of the 11th International Symposium on Superalloys , ed. R. C. Reed et al.(Warrendale,PA: TMS,2008), pp.443-451.
Dong Pan, Ph. D.candidate, Qingyan Xu, associate professor, Baicheng Liu, professor, are with the Key Laboratory for Advanced Materials Processing Technology, Ministry of Education, Department of Mechanical Engineering, Tsinghua University, Beijing 100084, China; Jiarong Li, research fellow, Hailong Yuan and Haipeng Jin, engineers, are with the National Key Laboratory of Advanced High Temperature Structural Materials, Beijing Institute of Aeronautical Materials, Beijing 100095, China. Prof. Liu can be reached at liubc@tsinghua.edu.cn.