




Sight distance is the continuous length of highway ahead visible to the driver. In design, two sight distances are considered: passing sight distance and stopping sight distance. Stopping sight distance is the minimum sight distance to be provided at all points on multi-lane highways and on two-lane roads when passing sight distance is not economically obtainable.
Stopping sight distance also is to be provided for all elements of interchanges and intersections at grade, including driveways.
Tab. 1 shows the standards for passing and stopping sight distance related to design speed.
Tab. 1 Sight distances for design
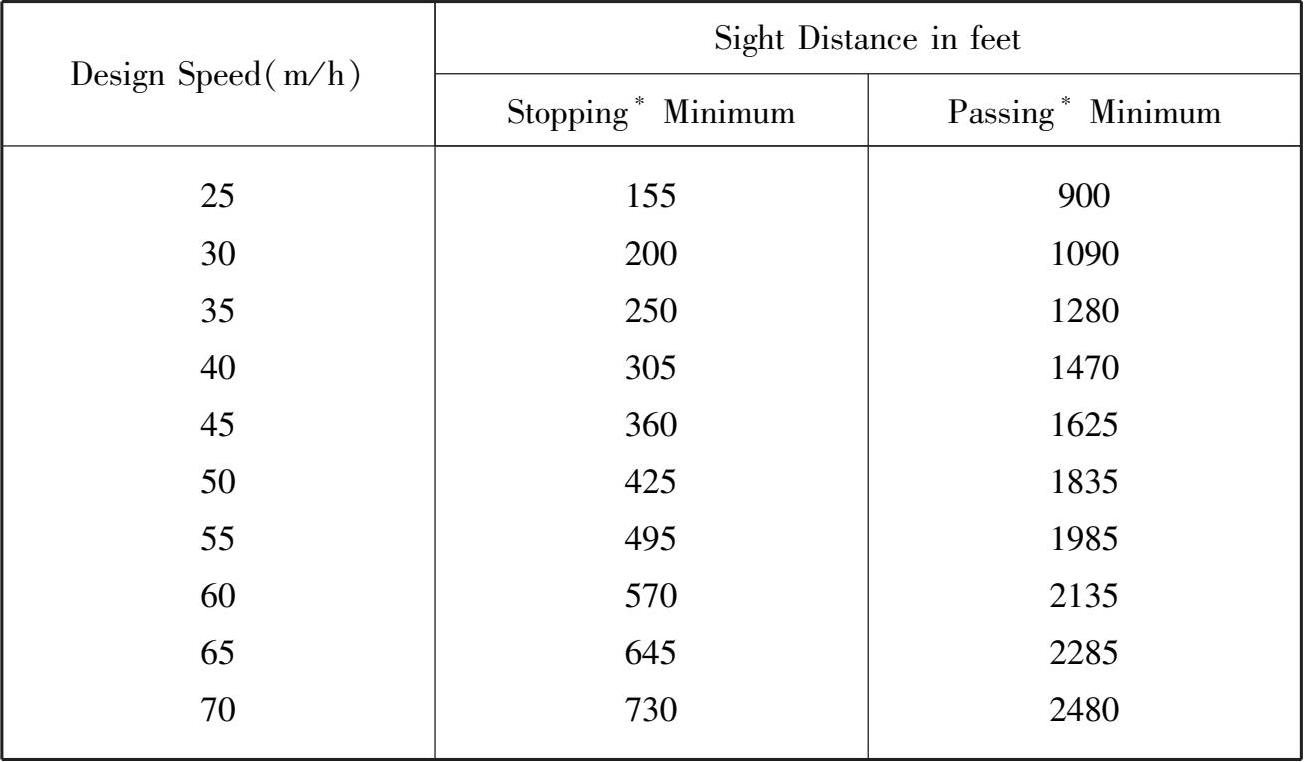
*Not applicable to multi-lane highways.
Passing sight distance is the minimum sight distance that must be available to enable the driver of one vehicle to pass another vehicle, safely and comfortably, without interfering with the speed of an oncoming vehicle traveling at the design speed, should it come into view after the overtaking maneuver is started. The sight distance available for passing at any place is the longest distance at which a driver whose eyes are 3.5 feet above the pavement surface can see the top of an object 3.5 feet high on the road.
Passing sight distance is considered only on two-lane roads. At critical locations, a stretch of four-lane construction with stopping sight distance is sometimes more economical than two lanes with passing sight distance.
The minimum stopping sight distance is the distance required by the driver of a vehicle,traveling at a given speed, to bring his vehicle to a stop after an object on the road becomes visible.Stopping sight distance is measured from the driver’s eyes, which is 3.5 feet above the pavement surface, to an object 2 feet high on the road.
The stopping sight distance should be increased when sustained downgrades are steeper than 3 percent. Increases in the stopping sight distances on downgrades are indicated in A Policy on Geometric Design of Highways and Streets, AASHTO, 2001.
Where an object off the pavement such as a longitudinal barrier, bridge pier, bridge rail,building, cut slope, or natural growth restricts sight distance, the minimum radius of curvature is determined by the stopping sight distance.
For sight distance calculations, the driver’s eyes are 3.5 feet above the center of the inside lane(inside with respect to curve) and the object is 2 feet high. The line of sight is assumed to intercept the view obstruction at the midpoint of the sight line and 2.75 feet above the center of the inside lane. Of course, the midpoint elevation will be higher or lower than 2.75 feet, if it is located on a sag or crest vertical curve respectively. The clear distance (M) is measured from the center of the inside lane to the obstruction.
The general problem is to determine the clear distance from the centerline of inside lane to a median barrier, retaining wall, bridge pier, abutment, cut slope, or other obstruction for a given design speed. Radius of curvature and sight distance for the design speed determine the middle ordinate (M) which is the clear distance from centerline of inside lane to the obstruction. When the design speed and the clear distance to a fixed obstruction are known, this figure also gives the required minimum radius which satisfies these conditions.
When the required stopping sight distance would not be available because of an obstruction such as a railing or a longitudinal barrier, the following alternatives shall be considered: increase the offset to the obstruction, increase the horizontal radius, or do a combination of both. However, any alternative selected should not require the width of the shoulder on the inside of the curve to exceed 12 feet, because the potential exists that motorists will use the shoulder in excess of that width as a passing or travel lane.
When determining the required middle ordinate (M) distance on ramps, the location of the driver’s eye is assumed to be positioned 6 feet from the inside edge of pavement on horizontal curves.
The designer is cautioned in using the values from Fig. 1 since the stopping sight distances and middle ordinates are based upon passenger vehicles. The average driver’s eye height in large trucks is approximately 120 percent higher than a driver’s eye height in a passenger vehicle. However, the required minimum stopping sight distance can be as much as 50 percent greater than the distance required for passenger vehicles. On routes with high percentages (10 percent or more) of truck traffic, the designer should consider providing greater horizontal clearances to vertical sight obstructions to accommodate the greater stopping distances required by large trucks. The approximate middle ordinate (M) required for trucks is 2.5 times the value obtained from Fig. 1 for passenger vehicles.
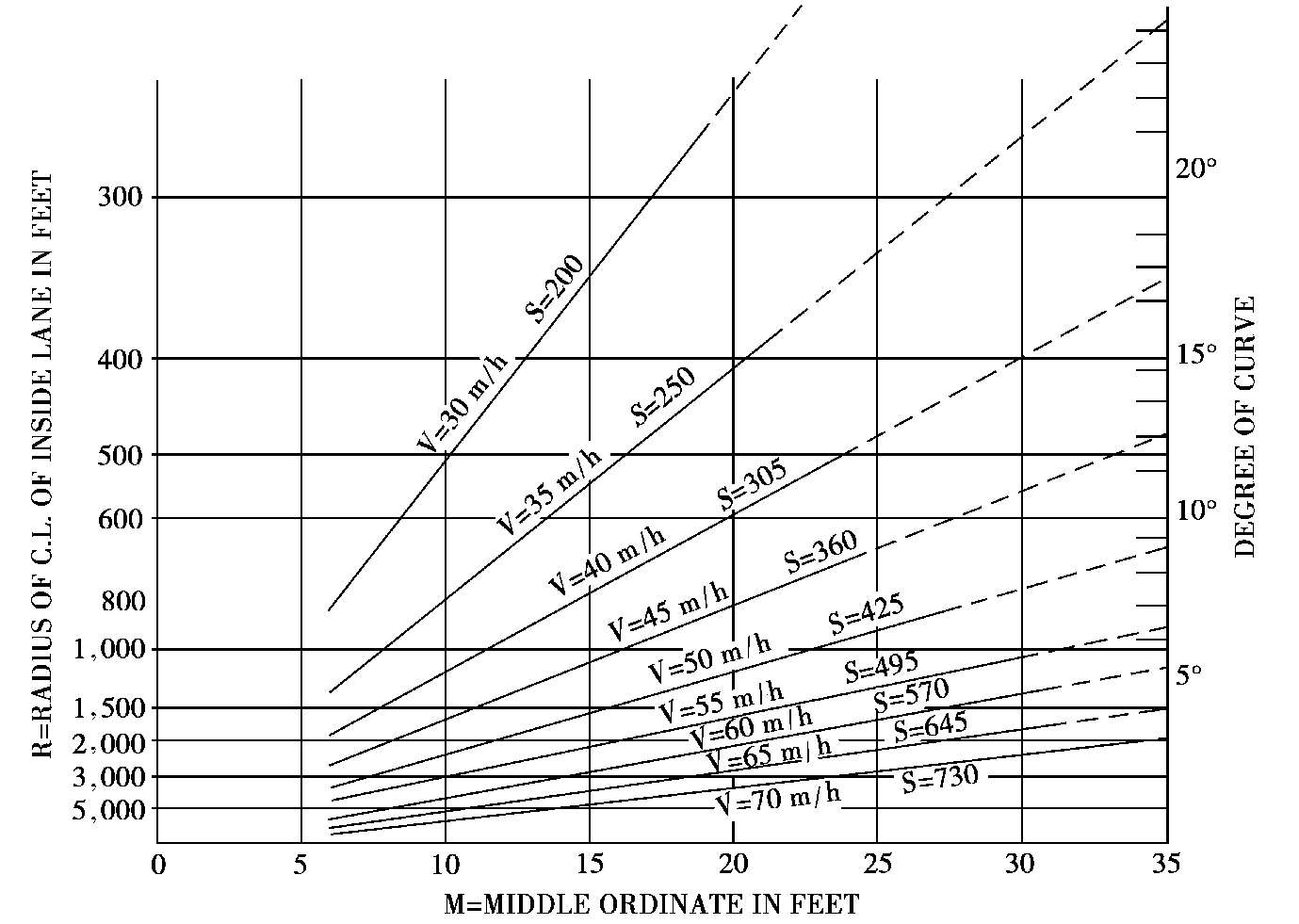
Fig. 1 Minimum stopping sight distance on horizontal curves
passing sight distance 超车视距
stopping sight distance 停车视距