




GMAT考试是对于那些希望上MBA的考生而设立的,而MBA要求学生具备很强的逻辑推断能力。Data Sufficiency题型恰恰适应了这一要求,做这类题目不仅要具备一般的数学基本知识,还要洞悉这类题目的Directions:
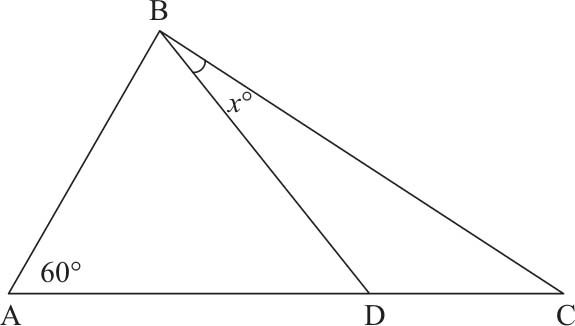
例1: In the figure above,what is the value of x?
(1)∠ABD equals 40°
(2)BD=CD
Directions:Each data sufficiency problem consists of a question,and two statements,labeled(1)and(2),that give data.You have to decide whether the data given in the statements are sufficient for answering the question.Using the data given in the statements plus your knowledge of mathematics and everyday facts(such as the number of days in July or the meaning of counterclockwise),you must indicate whether:

(A)Statement(1)ALONE is sufficient,but statement(2)alone is not sufficient to answer the question asked;
(单独1就足够回答问题,并且单独2不能够回答问题)
(B)Statement(2)ALONE is sufficient,but statement(1)alone is not sufficient to answer the question asked;
(单独2就足够回答问题,并且单独1不能够回答问题)
(C)BOTH statement(1)and(2)TOGETHER are sufficient to answer the question asked,but NEITHER statement ALONE is sufficient;
(1和2结合足够回答问题并且其中任一个单独都不能回答问题)
(D)EACH statement ALONE is sufficient to answer the question asked;
(1和2每一个单独都足够回答问题)
(E)Statement(1)and(2)TOGETHER are NOT sufficient to answer the question asked,and additional data specific to the problem are needed.
(1和2结合以及单独1和2都不能够回答问题,解答此问题需要额外的信息。)
Numbers: All numbers used are real number.
Figures: 1.A figure accompanying a data sufficiency problem will conform to the information given in the question,but will not necessarily conform to the additional information given in statements(1)and(2).
2.Lines shown as straight can be assumed to be straight and lines that appear jagged can also be assumed to be straight.
3.You may assume that the positions of points,angles,regions,and so forth,exist in the order shown and that angle measures are greater than zero.
4.All figures lie in a plane unless otherwise indicated.
上面第1点说明所伴有的图形将遵循题目中给出的信息,但并不必要地遵循statement(1)和statement(2)中给出的附加信息。这是什么意思呢?
比如说上面的例1中,statement(1)中说∠ABD=40°,但是图中没有必要把∠ABD就画成40度;statement(2)中说BD=CD,但是图中没有必要说画的时候让BD和CD相等。
我们来分析一下例题1:
单独1,∠ABD=40°无法解决问题;
单独2,BD=CD,只能够得到∠ACB=x°,也无法得到x的值;
把1和2结合起来考虑,得到:60+40+2x=180,那么显然能够求得x的值;
所以选择(C)。
从上面例1中我们也可以看出,在解Data Sufficiency的题目时,可以分为几步走:
第一步: 如果题目是文字描述题,并且有必要的话,列出相应的式子或者方程。
第二步: 首先考虑单独statement(1)和单独statement(2)是否充分,
如果只有statement(1)充分,那么选择(A);
如果只有statement(2)充分,那么选择(B);
如果两者分别都充分,那么选择(D)。
第三步: 如果单独statement(1)和单独statement(2)都不充分,那么把两者结合起来考虑是否充分。如果充分,选择(C);如果不充分,选择(E)。
例2: What is the value of a?
(1)a 2 +a-12=0
(2)|a|>2
解: 单独(1)得到a=3 or a=-4,有两个可能的值,因此单独(1)不能回答;
单独(2)显然无法确定a的值。
把(1)和(2)结合起来,许多同学轻易地就认为a=3,选择(C)。大错特错也,要知道a=-4,绝对值还是大于2,所以a还是有两个值,3和-4,这样结合起来也不能回答,因此答案为(E)。
在解Data Sufficiency题目时,有几点考生要正确理解,否则往往会浪费时间或选错选项。
第一点:不要浪费时间具体演算。
解Data Sufficiency题目,强调的是给出的信息是否足够回答问题,而不是要浪费时间去解题。不知大家是否看到例1中最后列出了一个方程60+40+2x=180,但是作者并没有去求解得到x等于多少?因为我们知道,从方程中能够求得x的值,已经回答了问题,至于具体值是多少,我们压根儿没有必要花费时间去求解。
在解应用性的Data Sufficiency题目时,大多数题目都需要考生列出方程,那么这些方程或者方程组有没有唯一的解,完全取决于方程的个数和未知数的个数是否相同,如果相同,那么往往就有解存在。
例3: How many hours were budgeted for a certain job?
(1)The number of hours budgeted for the job plus the actual number of hours used for the job was 140.
(2)The actual number of hours used for the job minus the number of hour budgeted for the job was 8.
解: 假设The number of hours budgeted for the job为x,the actual number of hours used for the job为y,那么得到:
单独(1)x+y=140,一个方程两个未知数有无数解
单独(2)y-x=8,一个方程两个未知数有无数解
把(1)和(2)结合起来考虑,两个未知数,两个方程,可以求解,所以答案为(C)。
当然,有时候需要注意:如果对某个未知数有特殊的限制条件,或者题目中所求的并不是具体未知数的值,那么有时方程个数小于未知数个数时也会有解,应该说这类题目在GMAT中还是比较常见的。
例4: If x and y are positive integers,what is the value of y?
(1)21x+20y=500
(2)x+y=24
解:
这道题目有很大的迷惑性,有些同学不加思考就选择(C),但是这样做忘了x,y都是正整数的限制。单独(1)得到
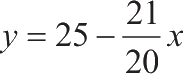 ,由于20和21互质,那么x必然是20的倍数,但是由于y也是正整数,所以必然取20,这样y的值也就确定,所以(A)为正确答案。
,由于20和21互质,那么x必然是20的倍数,但是由于y也是正整数,所以必然取20,这样y的值也就确定,所以(A)为正确答案。
第二点:答案唯一性原则。
GMAT官方的Directions关于值的唯一性是这么描述的:In data sufficiency problems that ask for the value of a quantity,the data given in the statements are sufficient only when it is possible to determine exactly one numerical value for the quantity.也就是说这个值必须唯一,如果从条件确定出来有两个值或者两个以上值,回答都是不充分的。
例5: Is x equal to 1?
(1)x 2 =1
(2)x 2 +x-2=0
解: 题目中问x=1吗?
单独(1),得到x=1 or x=-1,单独(1)能够回答问题吗?绝对不能,问一个量的值是多少,这个值必须是唯一的。现在x有两个值,当然不能够回答问题。
单独(2),得到x=1 or x=-2,和(1)的分析一样,单独(2)也不能够回答问题。
把(1)和(2)结合起来得到x=1,那么能够回答问题,选择(C)。
例6: What is the value of t?
(1)The average(arithmetic mean)of t 2 and 8t is -8
(2)
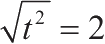
解: 单独(1),t 2 +8t=-8×2,即(t+4) 2 =0,因此t=-4,所以单独(1)能够回答问题;
单独(2)得到t=±2,t的值不唯一,因此单独(2)不能够回答问题;
所以(A)为正确答案。
第三点:答案充分性原则。
Data Sufficiency题目主要考查的是选项是否能够回答问题。在“answer the question”时,回答“Yes”是回答,回答“No”也是回答,只要能够回答就是答案。
例7: Is x equal to 1?
(1)x 2 =1
(2)x 2 -x-2=0
解: 题目中问x1吗?
从(1)中得到x=1 or x=-1,根据第二点答案的唯一性原则单独(1)不能够回答问题。
从(2)中得到x=2 or x=-1,尽管x有两个值,但是这两个值都不等于1,题目问x等于1吗?当然不等于。因此单独(2)就能回答问题,回答为“No”,所以此题正确答案为(B)。
第四点:条件独立性原则。
解Data Sufficiency题目是“answer the question”,而不是“solve the problem”。比如上面的例7,有些同学可能会这么解答。
既然(1)中x等于±1;(2)中x等于2或-1,那么可以求得x=-1,既然问x是否等于1,当然不等于,于是选择(C)。
这么做是有问题的。因为解Data Sufficiency题目的步骤是首先独立考虑两个条件,在考虑一个条件时绝对不能够受另一个条件的影响,如果一个条件已经能够独立回答问题,就绝对没有必要再把这两个条件联合起来求解。例7中单独(2)已经能够回答,那么刚才的做法就绝对不对。这也就是我们需要注意的:在选择(C)时应该仔细考虑是否可以选择(A)或者(B)。