




 Earth-Moon Mass Ratio from Mariner 9 Radio Tracking Data
Earth-Moon Mass Ratio from Mariner 9 Radio Tracking Data
S. K. Wong and S. J. Reinbold
Editor’s Note
The ratio of the mass of the Earth to that of the Moon is used to predict the evolution of the Moon’s orbit into the future. The ratio can be determined without any assumptions using radio tracking data from spacecraft. Here S. K. Wong and S. J. Reinbold do just that, using data from the Mariner 9 mission to Mars. They find the mass ratio to be 81.3007, very close to the currently accepted value. 中文
THE navigation of the Mariner 9 spacecraft from Earth to Mars was performed using phase-coherent range and doppler tracking data recorded by the Jet Propulsion Laboratory (JPL) Deep Space Network. These data also determine the Earth-Moon mass ratio, which involves the following physics: as the Earth revolves about the centre of mass of the Earth-Moon system, a sinusoidal curve is impressed on the range and doppler tracking data with a frequency equal to the sidereal mean motion of the Moon. This signature is shown in Fig. 1, where a perturbation of 0.0003 was made in the mass ratio (μ –1 =mass of Earth over mass of Moon). This sinusoidal variation in the tracking data can be eliminated by finding a value for μ –1 that properly represents the amplitude of the barycentre motion of the Earth. The procedure is direct and for all practical purposes is completely uncoupled from other parameters used in reducing the tracking data. 中文
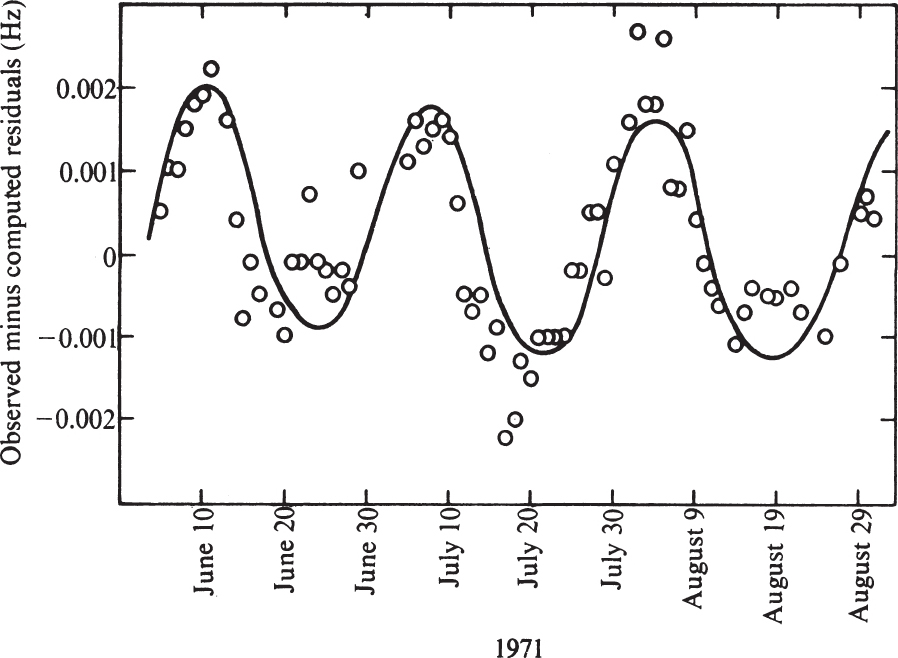
Fig. 1. The effect of Earth-Moon mass ratio on doppler residuals.
The mass ratio was determined from range and doppler data obtained over a period of 15 weeks (June 5 to September 15, 1971). The data coverage is shown in Table 1. We also show the statistics from the best determination. The data reduction was performed using the JPL Double Precision Orbit Determination Program 1 , which uses a Cowell integrated trajectory and a batch least squares filter. In weighting the range data, we have taken extreme care to assure optimal data utilization without conflicting with the doppler data. The Mariner Mars 1969 results showed that such conflicts can cause significant perturbations in the estimated parameters. 中文
Table 1. Tracking Data Statistics
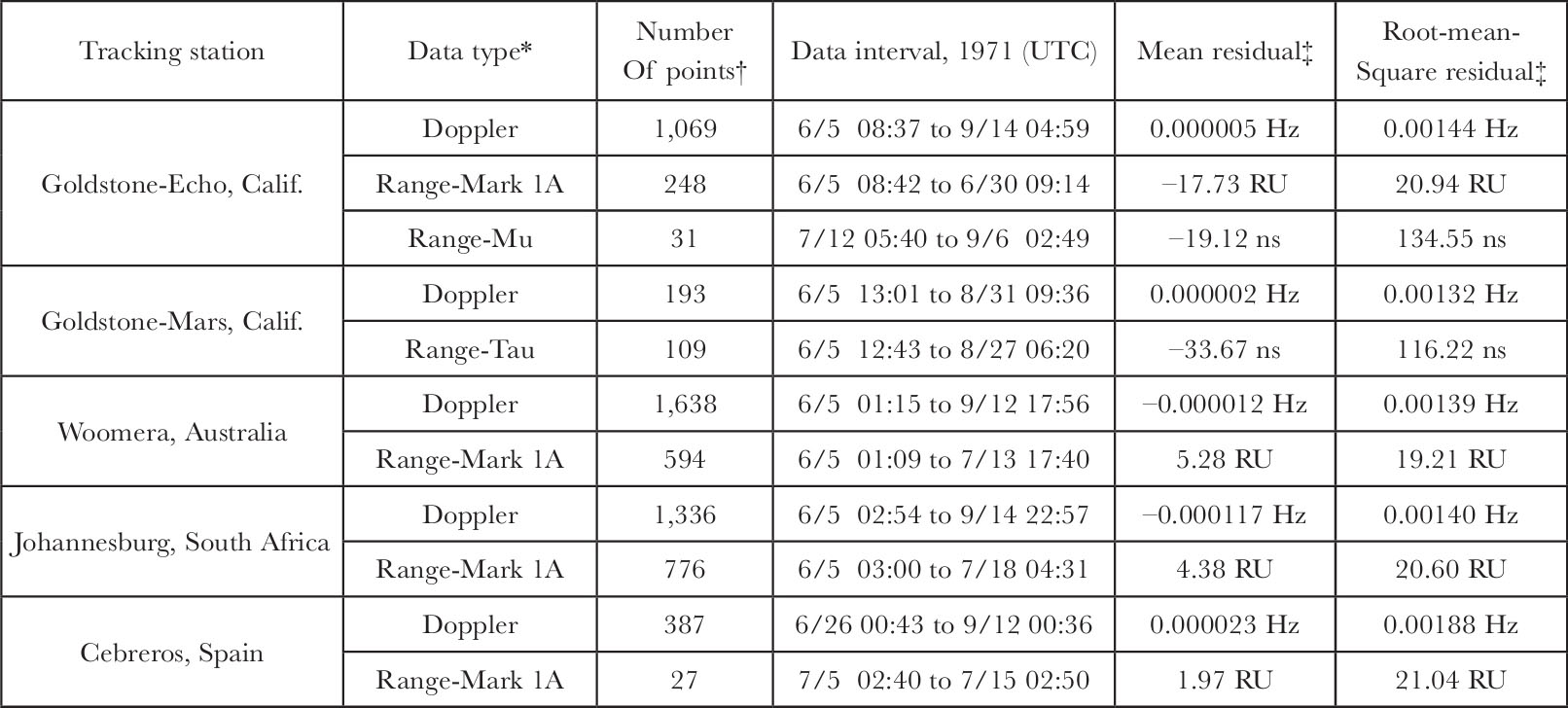
* Mark 1A=near-Earth ranging system; Tau and Mu=ranging systems using different ground hardware.
† Sample rate for doppler and range was 20 min.
‡ 1 Hz=65 mm/s; 1 RU (range unit) ≈ 1 m; 1 ns ≈ 0.15 m.
The Deep Space Network has three types of ranging systems: Mu, Tau, and Mark 1A. The Mu and Tau systems are capable of planetary distances, whereas the Mark 1A ranging system is limited to an effective one-way range of approximately 10 7 km. In weighting the range data the following factors were taken into consideration. First, assuming no external errors, the Mu and Tau systems are accurate to about 20 m. This includes system noise and transponder and ground equipment calibration errors. The Mark 1A ranging system is accurate to about 30 m. Second, because the radio signals travel through the ionosphere of the Earth and the interplanetary space plasma, there is a change in the radio signal path length. The group wave path length is increased, while the phase wave path length is decreased, corrupting both range and doppler measurements made from the radio signal. The charged particles of the Earth’s ionosphere could account for as much as a 15 m error in range; the charged particles in the interplanetary medium (space plasma) could account for as much as a 22 m error in range (J. F. Jordan et al ., paper presented at AAS/AISS Astrodynamics Conference, August 1970). Third, another possible cause of range error is the Z component of station location. This component is parallel to the Earth’s spin axis. The computed range value is sensitive to incorrect Z values when the probe declination becomes large in absolute value. An equation relating the two is 3
Δ ρ =Δ Z sin δ
Where ρ is the range datum and δ is the geocentric declination of the spacecraft. 中文
Because previous space mission data did not yield significant information on the Z component of station locations, JPL analysts used the Z values obtained by the Smithsonian Astrophysical Observatory (SAO) in 1969. The change in the Z component was as much as 56 m. Assuming that the Z values from SAO may be in error by 30 m and the maximum absolute value in geocentric declination for Mariner Mars 1971 is 29.15°, the above equation would yield a range error of 14.6 m. The doppler data are insensitive to this Z -component error. Even though the Z component of a station location is not too well determined, the distances from the spin axis and the longitude are known to better than 3 and 5 m, respectively. 中文
A number of solutions with different combinations of weights for each data type and different sets of estimated parameters were examined. The standard set of estimated parameters includes the probe position and velocity (6), solar pressure (3), attitude control leaks (3), station locations (15), and the Earth-Moon ratio (1). An a priori statistic of 0.0166 was applied to the mass ratio parameter. These solutions and their identifications are given as follow 中文
Case 1, Doppler only (doppler weight=0.015 Hz) with standard estimated parameter set. Case 2, Range only (range weight=100 m) with standard estimated parameter set. Case 3, Doppler and range (doppler weight=0.015 Hz, range weight=100 m) with standard estimated parameter set. Case 4, Doppler and range (doppler weight=0.015 Hz, range weight=100 m) with standard estimated parameter set plus Mars and Earth-Moon barycentre ephemeris parameters. Case 5, Doppler and range (doppler weight=0.015 Hz, range weight=50 m) with the estimated parameter set as in Case 4. 中文

All solutions yielded nearly the same mass ratio. Cases 1 and 2 give remarkable agreement on a mass ratio between the two data types. With such good agreement, the relative weight of the two data types becomes less significant. Cases 3 and 4 show that the lunar ephemeris error is probably too small to have an effect on the mass ratio estimate. Possible error sources are the periodic variations in the interplanetary medium. W. G. Melbourne has shown (12th Plenary Meeting of the Committee on Space Research, Prague, 1969) that a 28 day sinusoidal variation of solar flux of 0.1% could produce an error of about 0.001 in the mass ratio, but that it is not likely. Also, the agreement of mass ratios computed from the data gathered from several interplanetary spacecraft does not indicate this sort of systematic error unless the phase of the flux variation is the same for each mission, which does not seem likely. 中文
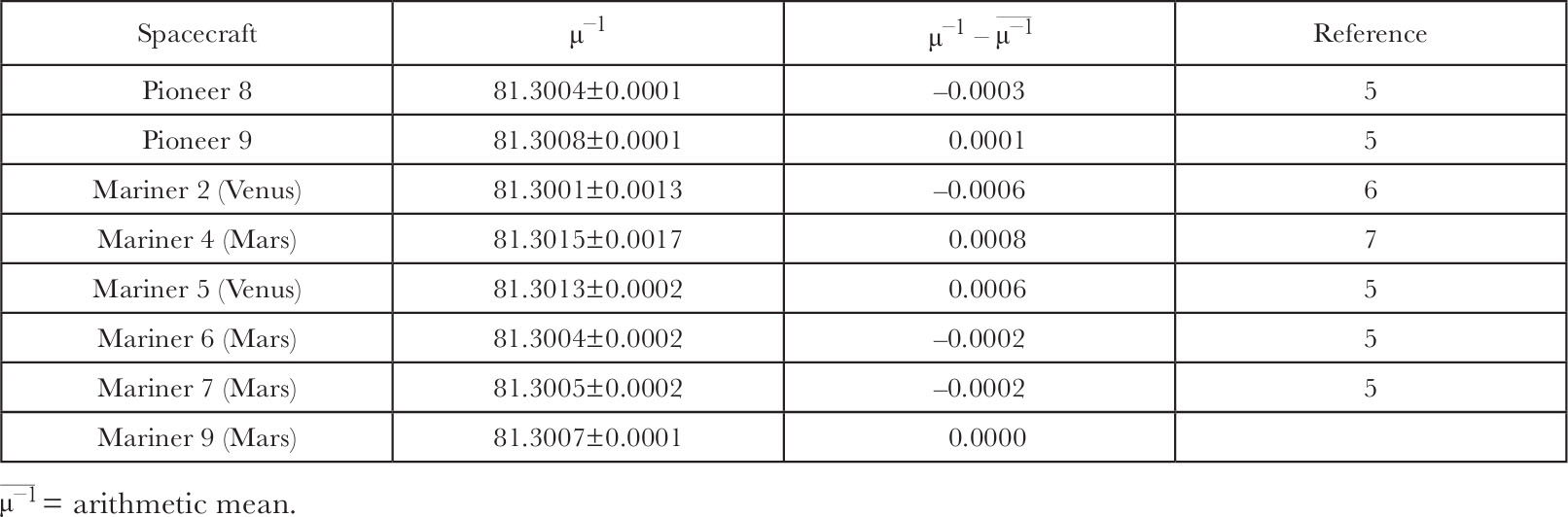
The results from the Mariner Mars 1971 data are given in Table 2 together with previous results obtained from Pioneers 8 and 9 and Mariners 2, 4, 5, 6 and 7. Values computed for Pioneers 8 and 9 and Mariner 2 were obtained from solutions using only doppler data. It is interesting to note that the Mariner 9 value and the mean of all spacecraft determined values of μ –1 are 81.3007. The deviations from the arithmetic mean of the Mariner and Pioneer values are tabulated in Table 1. Further, the mass ratio computed from the last five interplanetary spacecraft launched (Pioneers 8 and 9 and Mariners 6, 7 and 9) showed a spread of only 0.0004. The values for Pioneers 8 and 9 and Mariners 6, 7 and 9 are 81.3004, 81.3008, 81.3004, 81.3005 and 81.3007, respectively. This provides a good indication of the accuracy of μ –1 . The decrease in the fluctuation of the mass ratio value can be attributed to the improvement of data quality owing to changes in Deep Space Network tracking systems and the change in computer software from single precision to double precision. 中文
Table 2. Estimates of the Earth-Moon Mass Ratio, μ –1
We thank W. L. Sjogren for discussion and review of this article. This research was supported by NASA. 中文
( 241 , 111-112; 1973)
S. K. Wong and S. J. Reinbold
Jet Propulsion Laboratory, Pasadena, California 91103
Received September 11, 1972.
References: