




 On the Origin of Deuterium
On the Origin of Deuterium
F. Hoyle and W. A. Fowler
Editor’s Note
Deuterium is hydrogen that contains a neutron in its nucleus. It is relatively abundant in the universe, most having been created in the first few minutes after the Big Bang. Here Fred Hoyle and William Fowler investigate several ways in which it could also be created in astrophysical situations. The shock waves associated with supernovae (exploding old stars), and cosmic rays hitting clouds of gas can both generate deuterium, but not enough to explain the observations. The arguments advanced here were an attempt to avoid invoking a Big Bang at all, which Hoyle spent the later years of his life opposing. The Big Bang is, however, now the generally accepted explanation for the origin and properties of the Universe. 中文

The origin of deuterium has always been a problem for theories of stellar nucleosynthesis. A general solution is proposed and shown to be applicable under several astrophysical circumstances in the light of new observations of the Galactic abundance of deuterium. 中文

CESARSKY, Moffet and Pasachoff 1 have recently observed an absorption feature in the spectrum of radiation from the Galactic centre at 327.38837±0.00001 MHz. They interpret this as arising from the ground-state hyperfine transition in deuterium near 91.6 cm which is analogous to the well-known 21 cm line of ordinary hydrogen. If they assume the feature observed to be due to noise they are able to set an upper limit
D/H<5×10 –4
while an analysis assuming the feature to be due to deuterium yields
3×10 –5 <D/H<5×10 –4
(1)
These results are to be compared with the terrestrial value of 1.5×10 –4 and to the upper limit for the proto-solar value of 3×10 –5 (refs. 2, 3). 中文
Jefferts, Penzias and Wilson 4 report line emission from a cloud within the Orion Nebula at 144,828 MHz and attribute it to the J =2 to J =1 transition in DCN. In a separate investigation of the J =1 to J =0 transition at 72,414 MHz, the hyperfine components expected for DCN have also been found 5 , setting the identification beyond reasonable doubt. The cloud in question probably has dimensions of the order of a light year and a mass of order 10 2 M ⊙ , considerably less than the Orion Nebula itself. The H II region of Orion, which is the part seen optically, has mass ~10 3 M ⊙ , whereas a larger scale molecular cloud, detected in the 2.6 mm radiation of the CO molecule, has been estimated by Solomon (private communication) to have mass ~10 5 M ⊙ . 中文
The emission from the J =1 to J =0 transition is approximately as strong as that in 1 H 12 C 15 N and 1 H 13 C 14 N, so
D/H=6×10 –3
(2)
a remarkably high value. The ratio determined in this way applies to D and H in combination with CN, not to D and H in atomic form. Thus the D/H ratio in the interstellar gas could still be comparable with the terrestrial value, or with the value of 3×10 –5 referred to above. 中文
The position concerning deuterium has therefore changed from doubt concerning its widespread existence in the Galaxy to one in which it is a reasonable inference that D/H of order 10 –4 occurs on a large scale, and the problem of the origin of deuterium now seems more urgent than it did before. Together with R. V. Wagoner 6 , we found some years ago that significant quantities of D could arise in a low density Friedmann universe. Defining a parameter h from the relation (applicable after e ± -pair annihilation in the universe)

where ρ b is the baryon mass density and T 9 is the radiation temperature measured in units of 10 9 K, we found D/H comparable with the terrestrial ratio of 1.5×10 –4 when h ≃6×10 –6 . Setting T 9 =2.7×10 –9 K for the present temperature leads to ρ b ≃10 –31 g ml. –1 for the present baryon density, which is in good agreement with estimates which have been made of the average density of matter in galaxies by Oort 7 and Shapiro 8 . 中文
It is an unexpected feature of such a primordial mode of synthesis that the well-known cosmological parameter q 0 turns out to be small, and close to zero 6 , instead of the value 0.5 required to “close” the universe. Many cosmological investigations, the formation of galaxies for example, are much more awkward in hyperbolic models ( q 0 ≃0) than they are for q 0 ≥0.5. One might seek to evade the resulting difficulties by arguing that, unlike an idealized Friedmann model, the actual universe is inhomogeneous. It might be supposed that the initial state of the universe was very patchy, with h falling below 10 –5 in some places. On the other hand, the 2.7 K radiation background is exceedingly uniform, both locally and from one part of the sky to other distant parts of the sky. This uniformity, while not forbidding sufficiently fine scale inhomogeneities, would seem to us to constitute a warning against this line of argument. 中文
It is also important in the new circumstances of the problem to reconsider possible astrophysical modes of origin for deuterium. Should it turn out that D/H is locally variable, astrophysical processes would be preferred to primordial synthesis, but if the D/H ratio is found to be universal then primordial synthesis would be preferred. 中文
Investigations going back to the middle nineteen fifties have repeatedly shown that D is best produced astrophysically under non-thermodynamic conditions. We imagine α particles projected at high speed into an ionized gas composed mainly of hydrogen. If the gas temperature has some moderate value, say 10 5 K for definiteness, nuclear reactions leading to D production can occur. D can be knocked out of an α particle by a spallation reaction, and neutrons knocked out of the 4 He can subsequently be captured by protons of the ambient gas. For example, α particles entering gas at speed c /3 have a kinetic energy relative to the gas of ~200 MeV, which is 50 MeV per nucleon, and at such a bombarding energy the total cross section for all the reactions leading to D production is about 5×10 –26 cm 2 , that is, 50 mb according to Audouze et al. 9 . Although the stopping cross section due to Coulomb scattering is greater than this, it is clear that a significant fraction of the α particles will produce a D nucleus. Because the D is thus formed within a comparatively low temperature gas it is not subject to subsequent breakup, except in rare cases where it happens to be hit by a further incoming α particle. 中文
There are many ways in which this general idea can be used; for example, it can be applied to cosmic rays entering a cloud of gas. The production of D (and 3 He) through the spallation of 4 He was discussed a decade ago 10 in connexion with magnetic flares in the solar surface. The nuclear physics involved is largely independent of the acceleration mechanism. But processes involving cosmic rays are not capable of explaining large D concentrations of the kind that have now been reported. Cosmic rays are too wasteful of their energy in this respect. D production reaches the geometrical cross section and thus occurs most efficiently when the energy per nucleon is about 30 MeV. Energies as high as several GeV, which is where the main reservoir of cosmic ray energy lies, are not required. For greatest efficiency we must look therefore to processes involving speeds ~ c /3. 中文
Such speeds have indeed been found for shock waves generated by stellar explosions 11 . The shock wave starts in the region of the explosion at a speed not much different from the speed of sound, which is always much less than c . As it travels outwards into the lower density regions of the envelope, however, the wave speeds up. It is therefore in the outer envelope that speeds of the required order have been reported in previous investigations. 中文
The shock wave condition we have in mind can be applied much more generally than to a supernova. The basic requirement is for a supply of radiant energy (or of relativistic particles) to emerge from some local source into a diffuse outer envelope of gas containing 4 He. The larger the energy supply the better. For a supernova we expect ~10 50 erg to be available, which is much less than a case reported recently by Appenzeller and Fricke 12-14 . Their work is concerned with objects having masses between 10 5 and 10 6 M ⊙ —that is, masses of the order of the whole Orion Nebula. Under suitable conditions nuclear energy generation in a time scale of a few thousand seconds, yielding ~10 56 erg, can lead to expansion and disruption of the whole object. Because most of the energy is taken up in the radiation field, it is possible that a bubble of radiation, say with total energy ~10 55 erg, may work its way to the outer part of the object and may propagate thence into a surrounding diffuse cloud. Outbursts involving relativistic particles, also with energies of ~10 55 –10 56 erg, occur in radio galaxies. Indeed, outbursts from radio sources involving energies up to ~10 60 erg have been considered. 中文
The energy in question, whether radiant or in the form of relativistic particles, would escape from the source at speed c if it were not for the material of the surrounding envelope. But as the envelope becomes more tenuous the radiation is able to push material ahead of it at speeds which approach more and more to c . In such circumstances the material of the envelope is accelerated forward impulsively at a shock front, and nuclei present in it are subject to spallation if the shock becomes violent enough. Deuterium is then formed, either by direct spallation of 4 He or from neutrons coming from 4 He, the neutrons being captured subsequently by protons downstream of the shock. 中文
The properties of shock waves are usually investigated through the equations of continuum mechanics. The complex physical processes taking place at the shock front are idealized by a discontinuity, much as an impulse to a body is idealized in classical mechanics. We define v i , v s as velocity components normal to the shock, with subscripts i, s referring to conditions upstream and downstream of the front respectively. For simplicity, taking hydromagnetic effects to be small, and taking a frame of reference in which the front is stationary, we have the following conservation relations across the front:

where p is the pressure and u is the internal energy per unit mass. Terms in p i , u i are small in our case. Omitting them, we can satisfy equations (4) with
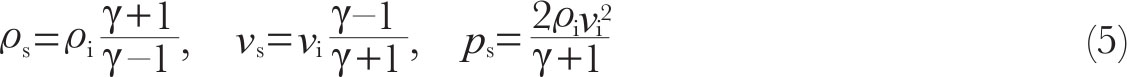
where
(γ–1) ρ s u s = p s
(6)
γ is the ratio of specific heats downstream of the shock, and is close to 4/3 in a radiation dominated problem. Putting γ=4/3 in equation (5) we get
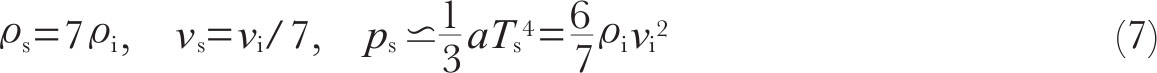
The third of these equations can be regarded as determining v i when T s and ρ i are given. The value to be used for T s depends on the energy supply, while ρ i is the density of the envelope upstream of the shock. From here on, we shall be concerned with situations in which T s is high enough in relation to ρ i for the resulting value of v i to be of order c /3. 中文
Material flowing through the front experiences a change of velocity v i – v s =6 v i /7, which for v i = c /3 is 2 c /7. If we now think of the material in terms of individual particles, instead of from the point of view of continuum mechanics, the particles experience a change in velocity of 2 c /7 as they pass from being upstream to being downstream of the shock. We have to think of the front as possessing a finite depth and of particles from upstream experiencing collisions as they pass through a finite shock zone. As a velocity of 2 c /7 is equivalent to a bombarding energy of ~40 MeV per nucleon we have a situation similar to that discussed above for α particles projected into a stationary gas. Thus 4 He present in material upstream of the shock will be subject to fragmentation as it passes through the front. 中文
The present situation is actually more favourable to fragmentation than the case of α particles projected into an ambient gas, because all particles in the shock zone have come from upstream and have therefore experienced collisions. About half of the bombarding energy will be transferred to electrons, but otherwise the bombarding energy will be retained as random motions of protons and α particles within the shock zone, and the α particles will therefore be subject to breakup over the whole of the time they are within the shock zone. 中文
Material may be considered to have flowed downstream of the shock when electrons have had sufficient time to radiate the kinetic energy they have acquired from the heavy particles. Radiation through bremsstrahlung occurs with cross section

where E is the electron energy in units of the rest mass. In our case the electron energies are ~20 MeV, and the logarithmic term in equation (8) is about 4, so the cross section is ~10 mb. This is about five times less than the spallation cross section. On the other hand, an electron of energy 20 MeV has a velocity about five times greater than a proton of the same energy. Hence
<σ v > Bremsstrahlung ≃<σ v > Spallation
(9)
from which it follows that an appreciable fraction of the α particles must be fragmented by the time they pass downstream of the shock. 中文
At this stage we have to distinguish two cases according to whether the gas density is high enough for neutrons from spallation to be captured by protons, or not. The former case is more efficient in its deuterium production by an order of magnitude. Considering this case, and taking the material of the initial cloud to have the usual helium concentration of ~0.25 by mass, and using say 40% for the fraction of the helium experiencing complete spallation, we arrive at a D concentration downstream of the shock of 0.1. Thus the mass density of D downstream of the shock is 0.1 ρ s . Using (7), and setting v i = c /3, we have
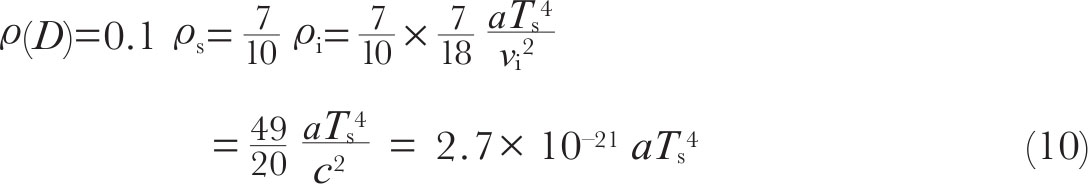
or
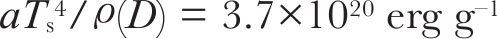
This is a relation between energy supply and deuterium production. The energy “cost” in the radiation field to produce one gram of deuterium is seen to be ~3.7×10 20 erg. So to produce an average D/H ratio equal to the proto-solar value of ~3×10 –5 throughout a mass M requires ~10 16 M erg, from which we see that to obtain D/H ~3×10 –5 throughout the Galaxy requires ~3×10 60 erg. Because this value is within the range that can be contemplated it seems possible for the present process to give a galactic deuterium concentration comparable to the initial solar system value. 中文
The present considerations require neutrons from the spallation of 4 He to be captured by protons. For this condition to be satisfied ρ i must not be much less than ~10 –8 g cm –3 . Otherwise the efficiency of D production is reduced by an order of magnitude, and the energy requirement is increased correspondingly. In the rest of this article we shall take ρ i =10 –7 g cm –3 for definiteness, and will consider the rates which are then operative for various processes. 中文
The first question to be asked is: what will be the order of the depth of the shock zone? Taking ~3×10 –7 g cm –3 as the average density within the shock zone, and noting that <σ v > Bremsstrahlung ≃3×10 –16 , we see that the electrons lose their energy in a time ~2×10 –2 s. In this time material flowing at a mean speed of say ~ v i /2= c /6 travels a distance ~10 8 cm. This gives the order of the depth of the zone. 中文
To prevent radiation downstream of the shock from simply streaming through the front it is necessary that the optical depth of material within the shock zone shall be greater than unity. For the numerical values considered in the previous paragraph there are about 30 g of material per unit area of the front. This is sufficient to dam back the radiation through Thomson scattering by the electrons. Before the radiation can penetrate the shock zone new material is then added from upstream. This circumstance does not depend on the particular numerical values used here. It depends essentially on the Thomson scattering for radiation being much larger than the bremsstrahlung cross section, ~10 –24 cm 2 compared with ~10 –26 cm 2 . 中文
Once bremsstrahlung transfers energy from the electrons to the radiation field other processes become involved, particularly Compton scattering. Because most of the bremsstrahlung energy from 20 MeV electrons consists of γ rays above 1 MeV, Compton scattering has a complex behaviour, certain scattering angles augmenting the radiation field, others transferring energy back to the electrons. A refined calculation would be necessary in order to consider such effects in detail. Here we shall simply take relation (9) to represent the order of magnitude of the relation of radiation losses to spallation. The conclusion from (9) is that an appreciable fraction of α particles are fragmented by the time they pass downstream of the shock. 中文
Deuterium produced within the shock zone will itself be subject to spallation, as will be 3 He and T. Consequently we shall not regard D production as being due to the immediate spallation of 4 He, but as arising from neutrons released in the breakup of 4 He. The lifetime of the neutrons against weak decay (~10 3 s) is very long compared to the time spent (~10 –2 s) in the shock zone. So the neutrons move downstream to regions where thermodynamic conditions can be considered to be established. Putting ρ i =10 –7 g cm –3 , v i = c /3 in (7) gives T s ≃ 7.6×10 6 K, which is far too low for deuterium formed by n+p→D+γ to be subject to spallation or to photodisintegration. The <σ v > value for this reaction is 7×10 –20 cm 3 s –1 , so for a hydrogen density ≃ 7×10 –7 g cm –3 downstream of the shock it takes some 30 s for the neutrons to be captured by protons. Because this is much less than the neutron half-life, although amply long enough for the neutrons to flow downstream well clear of the shock, we conclude that essentially all neutrons go to form D. 中文
To recapitulate to this point: Normal thermonuclear processes generate 4 He from hydrogen with very little production of deuterium. But if 4 He can be shaken loose into its constituent neutrons and protons in abnormal situations, such as those in the front of a high speed shock wave, conditions become favourable to D production provided the gas density is not so small that the neutrons decay before they are captured by protons. Conditions are then also favourable in that the temperature T ≃ 7.6×10 6 K behind the front leads to a lifetime for D(p, γ) 3 He of ~10 10 s, which is much longer than the time required for a local exploding object to disperse. The D/H ratio can be locally higher than the terrestrial value by as much as 10 2 , but after averaging with other exterior material the D/H value will be lowered. If the estimate of 3×10 –5 for the proto-solar value is typical of the interstellar medium as a whole, then the process described here could be the origin of all the deuterium in the Galaxy. Accompanying the production of D in the shock front will be that of a smaller amount of 3 He, perhaps enough to yield the proto-solar values (~10 –5 ) taken by Black 3 as typical. Further, because the explosion which produces the shock wave may well be due to nuclear energy generation converting a substantial fraction of hydrogen into helium, this process may also be the source of galactic 4 He. This brings us back (see pp. 23, 24 of ref. 6) full circle to the fact that conversion of hydrogen into helium in one part in four by mass yields the full energy of the background microwave radiation at 2.7 K and once again forces us to ask if there could have been a mechanism which provided the necessary thermalization. So far we have found no plausible affirmative answer to this question, but the coincidence of the numbers remains puzzling. 中文
We thank Dr Klaus Fricke for discussions on the production of shock waves in the implosion–explosion of massive objects, and the authors of refs. 1, 4 and 5 for informing us of their work in advance of publication. This work was supported in part by the National Science Foundation. 中文
( 241 , 384-386; 1973)
Fred Hoyle & William A. Fowler
California Institute of Technology, Pasadena, California
Received December 12, 1972.
References: