




 The Search for Signals from Extraterrestrial Civilizations
The Search for Signals from Extraterrestrial Civilizations
J. C. G. Walker
Editor’s Note
James Walker, an expert on the evolution of the terrestrial environment, here turns his gaze beyond the Earth’s atmosphere to consider the feasibility of detecting radio signals from extraterrestrial civilizations. This notion had been pursued experimentally since 1960, when astronomer Frank Drake performed a radio-telescope search. Drake devised an equation for estimating the probability of intelligent civilizations on other worlds. Walker combines a related estimate for the number of habitable planets with the detection capabilities of telescopes to deduce how long observations might need to proceed before an “intelligent” signal is found. The result is dispiriting: even with an optimistic estimate of how many inhabitable planets produce technologically advanced civilizations, it could take over a thousand years to spot them. 中文

Although the technology exists for exchanging radio messages with extraterrestrial civilizations, a successful search for such civilizations among the many stars that might support them could take more than a thousand years, even if most habitable planets are occupied by communicative civilizations. 中文

THAT the technology exists for sending and receiving radio messages over interstellar distances is not in doubt 1-5 so that, if there are similar technological civilizations based on stars not too distant from the Sun, we can, in principle, communicate with them. A problem, however, is to determine which star out of a large number of candidates is the home of a potentially communicative civilization 6-8 . The subject of this paper is the search problem of interstellar communication. 中文
Even the most optimistic estimates of the frequency of occurrence of potentially communicative civilizations suggest that a large number of stars will have to be searched before a civilization is encountered. If we let P c be the probability that a given star has a communicative civilization, we may write
P c = f P HP
(1)
Where P HP is the probability that the star has a habitable planet in orbit around it, and f is the fraction of habitable planets with communicative civilizations. This fraction involves the probability that a communicative civilization will evolve on a habitable planet as well as the average lifetime of communicative civilizations 6,9-11 . 中文
It may be impossible to determine the value of f by other than empirical means, but P HP is a quantity that can, in principle, be estimated from a knowledge of cosmogony and planetology. Dole 12 , for example, has made a detailed estimate of the probabilities that planets on which Man could exist are in orbit about stars of different spectral classes. His considerations lead him to conclude that P HP achieves a maximum value of 5.5% for stars of classes G0 to G4, and that 3.7% of stars in classes F2 to K1 have habitable planets; P HP is zero for stars outside this range. Dole’s estimates involve many assumptions, and improved values of P HP will undoubtedly become available in time, but his values are adequate for present purposes. They show, first, that several tens of stars must be searched, even if f is of order unity and, second, that we can estimate P HP for different stars and therefore can use this information to guide our search. 中文
One approach to the search problem is to assume that the other civilization will do most of the work, which implies that the search is limited to “supercivilizations” able to transmit detectable signals in all directions all the time 5,13 . We could not do such a thing 14 , for the power requirement of an isotropic call signal detectable at a range of 100 light year is approximately equal to the world’s present total power consumption 2,15 . We can signal over interstellar distances only by using a large radio telescope to concentrate the radiated energy into a narrow beam. It would be possible to use a number of transmitters to send continuous signals to an equal number of target stars, but for such a strategy to have a reasonable chance of success, the number of transmitters would have to exceed
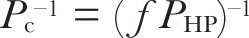
This number is larger than 18, using Dole’s values of P HP , and it may be very much larger, since f may be small. 中文
Even if there were several interstellar transmitters—and there are not—a strategy of continuous transmission to a select group of stars would not, however, be optimal. So as f is unknown, the probability of success for the transmitting civilization is proportional to the number of stars called regularly. In order to call the largest possible number of stars for a given level of effort, transmitter time must be shared among different target stars. How often, then, should a signal be sent to a given star 中文
Von Hoerner 6 has analysed this problem in general terms, pointing out that it is necessary to develop an optimal search strategy for both transmitter and receiver, and then to assume that the target civilization will perform the same analysis and arrive at the same conclusion. I present here a strategy for which the probability of success can be evaluated, at least as a function of f . 中文
An optimal search strategy should use all the information that we share with the target civilization. This includes the spectral classes of candidate stars and thus the values of P HP ; it includes the optimal spectral region in which to work, the region where unavoidable background noise is minimal 1-5 and the distances to the candidate stars. 中文
This last quantity provides the only indication we have as to how often we should look at any given star. The natural repetition period 8 for a star at distance R is

where c is the velocity of light. It is the time for a contact signal to travel to the star and for a reply to return. I shall assume that the transmitter sends a contact signal every T years to every star within range having a non-zero P HP . Excess transmitter capacity would be used to increase the range of the search rather than to provide more frequent contact signals. 中文
Although there are a number of ideas about the wavelength to use for contact signals 1,5 , a search in frequency cannot be eliminated entirely because extremely narrow bandwidths must be used for interstellar communication 2 . I shall not consider the frequency search explicitly, so for simplicity in the analysis I shall assign this task to the transmitter. Thus the contact signal to be sent out every T years will sweep slowly over the optimal spectral region, and the receiver may confine its search to a single frequency. 中文
With the transmitter strategy thus defined, it is possible to determine the optimal receiver strategy and evaluate the rate of success. From the point of view of the receiver, let us redefine P c to be the probability that a given star has a civilization that sends a contact signal to the receiving star every T years at the wavelength on which the receiving civilization is listening. This redefinition introduces a corresponding change in the definition of the unknown fraction f , but no change in the known probability P HP . 中文
If, at the beginning of the search, the receiver devotes a period of time ∆τ to listening to a given star, the probability that it will receive a call is
P s = P c ∆τ/ T
(3)
The rate of success in the search is therefore f P HP / T yr –1 , where P HP / T depends on the spectral class and the distance of the target star and f is the same for all stars. Because the repetition period T increases linearly with distance to the target star, the success rate is highest for the closest stars. Using Dole’s figures 17 , we find a success rate for α Centauri of 1.3×10 –2 f yr –1 ; for ε Eridani and τ Ceti the success rates are both about 1.5×10 –3 f yr –1 . For a G0 star at 100 light year, however, the success rate is 2.7×10 –4 f yr –1 . 中文
But the receiver should not devote all its time to the closest star. This star may not be the home of a communicative civilization. After the receiver has devoted a large number n of randomly spaced listening periods ∆τ to a given star, the probability that the receiver will have failed to receive a call, assuming that there is a transmitter associated with that star, is exp ( –n ∆τ/ T ). The probability of success on the next look at the star is therefore
P s (τ)= f P HP (∆τ/ T ) exp (–τ/ T )
(4)
where τ= n ∆τ. 中文
The optimal receiver strategy is now clear. The success rate is maximized if each listening period ∆τ is devoted to the star for which ( P HP / T ) exp (–τ/ T ) is greatest. As the search progresses, the number of stars included in the search increases steadily, for each star within range the value of τ increases, and the instantaneous success rate grows steadily smaller. Each try, however, adds the greatest possible amount to the cumulative probability of success. How much time, on average, must elapse before success is achieved 中文
Suppose that there are N i stars of spectral class i per unit volume and let P HP ( i ) be the probability that each of these stars has a habitable planet. After a total time t has been devoted to the search, following the strategy outlined above, the cumulative probability of success is

where ε is the instantaneous success rate at time t given by

From Dole’s Table 18,
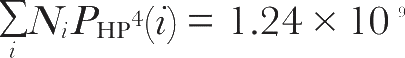 per cubic light year, so
per cubic light year, so

and

where t is expressed in years. 中文
On the average, contact will be achieved when P s =1 or after a search that has lasted
t 0 =1,380 f –4/ 3 yr
(9)
Values of t 0 corresponding to several assumed values of f are shown in Table 1. Also shown is the average distance that separates communicative civilizations for these values of f . 中文
Table 1. Search Strategies for Various Distributions of Civilizations
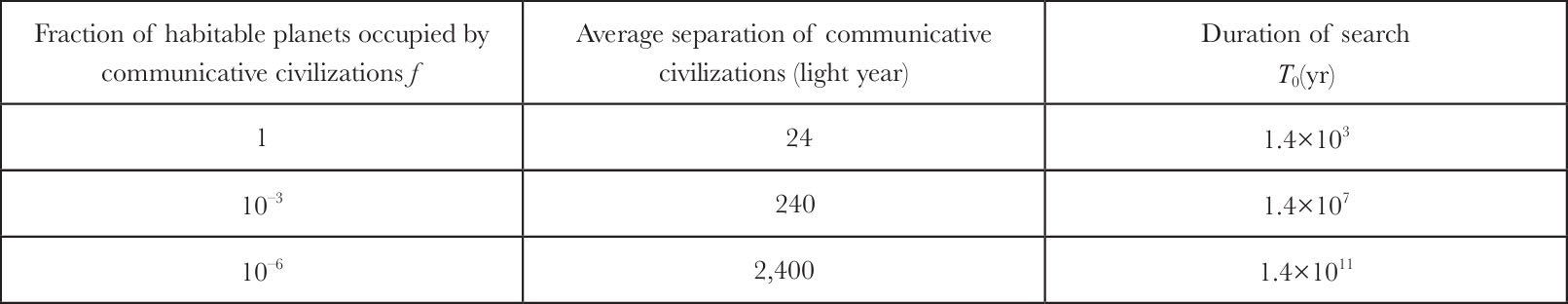
We see that even with optimistic assumptions concerning the frequency of occurrence of communicative civilizations, the time required for a successful search is long. Of course, t 0 is, strictly speaking, telescope time devoted to the search, not total elapsed time. The duration of the search is therefore inversely proportional to the number of receiving telescopes and could be shortened by a massive effort. Alternatively, it is possible that the transmitter strategy I have assumed is incorrect, and that more frequent calls would be optimal, say m calls every T years. In this case the duration of the search differs from the values in Table 1 by a factor of 1/ m , provided the receiving civilization knows the value of m . 中文
The conclusion, therefore, is disappointing. If every habitable planet has a communicative civilization there might be 50 such civilizations within 100 light year of us 18 . We possess the technology to exchange messages with this multitude of other worlds, if only we can find them. Unless my assumed transmitter strategy is seriously in error, however, or unless habitable planets are substantially more abundant than Dole has concluded, the problem of finding the other worlds is overwhelming. These circumstances may limit us to a search for supercivilizations 16 . 中文
This research has been supported, in part, by a NASA grant. 中文
( 241 , 379-381; 1973)
James C. G. Walker
Department of Geology and Geophysics, Yale University, New Haven, Connecticut 06520
Received January 5; revised September 11, 1972.
References: